Imagine if
t,o = 1.02 (2% above critical). This is equivalent to the Universe expanding at 1% below it's Newtonian escape velocity. This is also equivalent to launching a rock from the Earth's surface at 1% below vesc (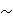
10 km/s). This rock will just reache the moon before turning around and falling back down.
Imagine the Earth 100× smaller (diameter 120 km) with the same mass. vesc is now 100 km/s and you must launch the rock at 1/100 % below vesc to just reach the moon. For 1% below vesc, the rock only reaches 2000 km before turning around. This is equivalent to cosmic expansion when a = 1/100 (z = 101, t = 17.4 Myr).
Now imagine the Earth 108× smaller, orange size, with vesc = 100,000 km/s. To just reach the moon, you must launch at 10-8% below vesc -- that's 1 cm/s slower than 1/3 light speed! At 1% too slow, the rock climbs 3 meters and turns around in 1 µs. At 1% too fast, and the rock flies past the moon at 1000 km/s. This is equivalent to 40 minutes after the big bang (z ~ 108).
Clearly, for the Universe to get so large from something so small, and be close to expanding at the escape speed at late times, it must have been "launched" at almost exactly the escape speed. This must be true for every galaxy (rock). Clearly, a simple "explosion" metaphor is very misleading, with its chaotic initial velocities.
Figure from Whittle's Teaching Company Course, and upcoming undergraduate text.