Selection of Homework Questions



Topic 8: Theory II : Stellar Dynamics
(1) Potential-Density Pairs
- Use Poisson's equation in spherical polar form to show that Jaffe's (1983) spherical density distribution:
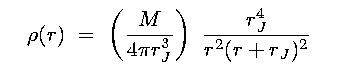 |
(Q8.1) |
gives the potential:
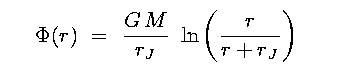 |
(Q8.2) |
Where M and rJ are constants.
- Verify that the total mass is M.
- Show that the circular speed is roughly constant for r
 rJ and decreases as r-½
for r
rJ and decreases as r-½
for r  rJ (B&T-1 Q 2.3)
rJ (B&T-1 Q 2.3)
(2) Potential Energy
- Show that the Gravitational potential energy of a spherical system
can be written:
 |
(Q8.3) |
where M(r) is the mass interior to radius r (B&T Q2.2).
- Evaluate this for a uniform density sphere of radius R.
- Approximate a SN progentior
star as a small (R 1.4 M
 core of radius
104km plus a 20 M
core of radius
104km plus a 20 M envelope of
radius 1AU, each of uniform density. Calculate the binding energy of
just the envelope.
envelope of
radius 1AU, each of uniform density. Calculate the binding energy of
just the envelope.
- If the core collapses to form a neutron
star of uniform density and radius 10km, and 1% of the gravitational energy
released is dumped into the envelope (99% escapes as neutrinos), can
the core collapse jettison the envelope?
- If it can, what is the velocity of the ejected envelope material (assuming
it all moves radially at the same velocity) ?
(3) Power Law Cores, and the Jeans Equation :
The goal of this problem
is to explore the behaviour of the
velocity dispersion near the center of a spherical non-rotating galaxy.
At radii r < ro assume that the density has the power law
form  (r) =
(r) =  o(r/ro)-
o(r/ro)- , with 0 <
, with 0 <  < 3.
Assume that the velocity
dispersion is isotropic at all radii and equal to
< 3.
Assume that the velocity
dispersion is isotropic at all radii and equal to  o at ro.
o at ro.
- Why is the constraint
 < 3 necessary ?
< 3 necessary ?
- Use the Jeans equation in spherical form to derive an expression for
the dispersion profile
 2(r) for r < ro
2(r) for r < ro
- For what range of
 does
does
 2(r)
2(r)  0 as r
0 as r
 0 ?
0 ?
- For what range of
 does
does  2(r) diverge as r
2(r) diverge as r  0 ?
0 ?
- For what value(s) of
 is
is
 2(r) independent of r as r
2(r) independent of r as r  0 ?
0 ?
- For the latter situation, what value of
 o (expressed in terms of
o (expressed in terms of
 o, ro, G) makes
o, ro, G) makes  independent of r at all r ? Evaluate this for the case in which
independent of r at all r ? Evaluate this for the case in which  o = 100
M
o = 100
M pc-3 and ro=100 pc.
pc-3 and ro=100 pc.
(4) Central Mass to Light Ratios :
Print out the pdf figure here (
link) which contains light
profiles for three elliptical galaxies (taken from Lauer et al). The units
for µV are
mag/ss in the V band. The central line-of-sight velocity
dispersions in these galaxies are :  (N1400) = 265 km/s;
(N1400) = 265 km/s;
 (N2832) = 330 km/s;
(N2832) = 330 km/s;
 (N3608) = 195 km/s. Assuming that
the galaxies are spherical and the velocity dispersion is isotropic and
the core is approximately isothermal, use "King's Method" to find the
core mass-to-light ratio of each galaxy in solar units. (Note that the
physical scale is plotted along the TOP axis; and think how core radius
is defined in terms of central surface brightness).
(N3608) = 195 km/s. Assuming that
the galaxies are spherical and the velocity dispersion is isotropic and
the core is approximately isothermal, use "King's Method" to find the
core mass-to-light ratio of each galaxy in solar units. (Note that the
physical scale is plotted along the TOP axis; and think how core radius
is defined in terms of central surface brightness).
(5) Relaxation Times :
Estimate the 2-body relaxation time in the following systems :
- The galactic bulge, which we approximate as a singular isothermal
sphere with circular speed Vc = 200 km/s containing stars of mass
0.6 M
 . The relaxation time should be given
as a function of radius. At what radius is the relaxation time equal to
1010 years?
. The relaxation time should be given
as a function of radius. At what radius is the relaxation time equal to
1010 years?
- A typical open cluster, with median radius 2 pc, mass 250
M
 , and stellar mass 1
M
, and stellar mass 1
M .
.
- The core of the
clobular cluster M4, with core radius 0.5 pc and central surface
brightness 17.88 mag/ss in V. You may assume that the typical
stellar mass is 0.6 M
 and the mass-to-light ratio is 1.6
M
and the mass-to-light ratio is 1.6
M /L
/L .
.
(6) Conceptual Question on DFs :
- Systems of stars can be described by a 7-dimensional distribution function, DF or just f. What are those 7 dimensions and what, exactly, does the DF describe? What, in qualitative terms, is the
form of the velocity portion of the DF for (i) stars at the galaxy center; (ii) stars in the solar neighborhood?
- Write down the collisionless Boltzmann equation (CBE) for f, and briefly discuss each term.
Why must physically plausible DFs also be solutions to the CBE? In other words, what does the CBE
describe about a system of stars and the nature of the DF?
- Imagine you are living "on" a star which is caught in a galaxy merger. Although your trajectory
in physical space may hurl you through dense bulges or sparse halos, your trajectory through the 6-D
position-velocity phase space keeps you moving along a path of constant stellar density.
Why is this?
- For a static potential, why is a distribution function with simple form f(E) automatically a solution
of the CBE, where E is the energy at a particular point in position-velocity phase space? What kind of
potentials would support DFs of the form f(E,|L|) and f(E,|L|,Lz)?
- Describe, in conceptual terms, how the CBE is "processed" to yield an observationally more
accessible equation: the Jeans equation? What properties of a stellar system does the Jeans equation
describe?
- Write down the Jeans equation for a spherical galaxy or star cluster. How do astronomers use
the Jeans equation to derive the mass distribution in a spherical non-rotating elliptical galaxy.
What basic observations and assumptions must be made, and how can higher quality observations help
inform those assumptions?




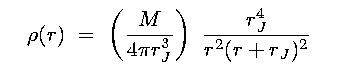
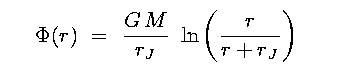
 rJ and decreases as r-½
for r
rJ and decreases as r-½
for r  rJ (B&T-1 Q 2.3)
rJ (B&T-1 Q 2.3)

 core of radius
104km plus a 20 M
core of radius
104km plus a 20 M envelope of
radius 1AU, each of uniform density. Calculate the binding energy of
just the envelope.
envelope of
radius 1AU, each of uniform density. Calculate the binding energy of
just the envelope.
 (r) =
(r) =  o(r/ro)-
o(r/ro)- , with 0 <
, with 0 <  < 3.
Assume that the velocity
dispersion is isotropic at all radii and equal to
< 3.
Assume that the velocity
dispersion is isotropic at all radii and equal to  o at ro.
o at ro.
 < 3 necessary ?
< 3 necessary ?
 2(r) for r < ro
2(r) for r < ro
 does
does
 2(r)
2(r)  0 as r
0 as r
 0 ?
0 ?
 does
does  2(r) diverge as r
2(r) diverge as r  0 ?
0 ?
 is
is
 2(r) independent of r as r
2(r) independent of r as r  0 ?
0 ?
 o (expressed in terms of
o (expressed in terms of
 o, ro, G) makes
o, ro, G) makes  independent of r at all r ? Evaluate this for the case in which
independent of r at all r ? Evaluate this for the case in which  o = 100
M
o = 100
M pc-3 and ro=100 pc.
pc-3 and ro=100 pc.
 (N1400) = 265 km/s;
(N1400) = 265 km/s;
 (N2832) = 330 km/s;
(N2832) = 330 km/s;
 (N3608) = 195 km/s. Assuming that
the galaxies are spherical and the velocity dispersion is isotropic and
the core is approximately isothermal, use "King's Method" to find the
core mass-to-light ratio of each galaxy in solar units. (Note that the
physical scale is plotted along the TOP axis; and think how core radius
is defined in terms of central surface brightness).
(N3608) = 195 km/s. Assuming that
the galaxies are spherical and the velocity dispersion is isotropic and
the core is approximately isothermal, use "King's Method" to find the
core mass-to-light ratio of each galaxy in solar units. (Note that the
physical scale is plotted along the TOP axis; and think how core radius
is defined in terms of central surface brightness).
 . The relaxation time should be given
as a function of radius. At what radius is the relaxation time equal to
1010 years?
. The relaxation time should be given
as a function of radius. At what radius is the relaxation time equal to
1010 years?
 , and stellar mass 1
M
, and stellar mass 1
M .
.
 and the mass-to-light ratio is 1.6
M
and the mass-to-light ratio is 1.6
M /L
/L .
.