Selection of Homework Questions

   

Topic 5: Spirals

An S0 galaxy has two components: (a) a spherical de Vaucouleurs R^¼ law bulge with effective radius R_e = 0.5 arcmin, and surface brightness µ_e = 20.5 mag/ss; and (b) an exponential disk whose major axis yields a scale length R_d = 1.15 arcmin and extrapolated central surface brightness µ(0) = 20.6 mag/ss. The disk is inclined by 60 degrees to the plane of the sky (i.e. 0 degrees is face-on).

a) Plot the observed major and minor axis surface brightness profiles for the bulge, disk, and total light (all on the same plot; surface brightness in mag/ss from 30 to 14 vs angular scale in arcminutes from 0 to 9). For the major axis, show where light is contributed by the bulge and disk in the ratios 1:5, 1:1, 5:1.

b) For both plots, show a typical B-band surface brightness for a dark night sky, and the "limiting radius" corresponding to a surface brightness µ = 26.5 mag/ss. What is the minor to major axis ratio measured at this limiting surface brightness? Why does this not naively yield an inclination of 60 degrees?

c) The total flux from the de Vaucouleurs bulge is L_tot 7.22 R_e² I(R_e). What is L_tot for a face-on exponential disk with central surface brightness I(0) [do the integral]? By what factor does a dust-free disk appear brighter when it is tilted by i degrees from face-on? Hence, for our galaxy disk, what is the face-on central brightness, µ_c(0), and what is the integrated bulge to disk flux ratio?

(2) 2-D Velocity Fields :

a) For a flat disk, derive the coordinate transformations to go from: R, to x', y' in the galaxy plane; and then to x, y in the sky plane after the galaxy is tipped by an angle i away from face on, such that the projected major axis is along the x axis.

b) What is the projected Doppler velocity for a point in the galaxy disk with circular velocity V_c and radial velocity V_r (+ve away from the nucleus), both in the plane of the galaxy?

c) On a simple 200 x 200 cartesian computational grid, use a contouring routine to generate "spider diagrams" (iso-projected velocity contours at intervals of 20 km/s) for the following velocity fields for a circular galaxy of radius 100 units, tipped through 60^o (label or color-code the contours, and indicate the zero velocity contour, which defines the kinematic minor axis) :

1. V_c = 150 (km/s) at all radii; V_r = 0 at all radii
   
2. V_c = solid body to R = 30, then flat at 150 km/s beyond; V_r = 0 at all radii
   
3. V_c = solid body to R = 30, then linear rise from 150 to 200 km/s; V_r = 0 at all radii
   
4. V_c = solid body to R = 30, then linear fall from 150 to 100 km/s; V_r = 0 at all radii
   
5. As for 4 but adding a uniform outflow of V_r = +50 km/s at all radii
   

d) For each of the velocity fields above, plot the integrated HI profile assuming that the galaxy fits within the HI telescope beam and has uniform surface HI density out to R = 100, but none beyond.

(3) Tully Fisher Relation :

a) Briefly outline a theoretical justification for expecting a relation between the luminosity of spiral galaxies and their rotation amplitudes of the form L V⁴   (note: the same justification applies for the Faber-Jackson relation for spheroids: L ⁴) . What additional constraints must hold for this relation to be valid?

b) A spiral galaxy with redshift cz = 7080 km/s has inclination i = 45^o, corrected apparent magnitude B_T^o,i = 13.3, and observed (semi-) rotation speed V_rot = 180 km/s. What's the true inclination-corrected full rotation amplitude, W? Use the Tully-Fisher relation M_B = -7.41(log W - 2.5) - 20.04 to infer the galaxy's absolute magnitude, M_B, and hence its distance modulus, and distance in Mpc. Adopting H₀ = 72 km/s/Mpc, what is the peculiar velocity of this galaxy?

c) If the optical rotation curve extends to 1.2 arcmin, use the Newtonian relation M(< R) =  R V² / G with geometry factor = 0.7, to estimate the mass-to-light ratio out to 1.2 arcmin. Is this reasonable for a typical spiral stellar population? If HI observations extend this velocity measurement out to 8 arcmin, what's the new mass-to-light ratio (use = 1.0 for a spherical halo)? Is this reasonable for a typical stellar population?

For an exactly edge-on pure stellar disk in which the stars all follow circular orbits at speed V_c, and in which the density of stars decreases with radius as n(r) r^-2, show that the line-of-sight velocity dispersion (LOSVD) is given by : F(V_los (V_c² - V_los²)^-½ in the range 0 < |V_los| < V_c and zero otherwise. Calculate < V_los >; _los; 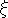₃; and 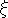₄ for such a disk.