Selection of Homework Questions



Topic 15: AGNs & Quasars
(1) Narrow Line Region (NLR) Gas Properties
Let's follow a path from typical spectroscopic observations of an AGN to deducing
a number of physical properties of its NLR.
Spectra taken through a
2 arcsec circular aperture show an H flux of 1.0×10-14 erg s-1 cm-2. The [OIII]5007
emission line has
a central wavelength of 5200A and a width (FWHM) of 10A. The
following flux ratios are measured : [OIII]5007/[OIII]4363 = 100 ;
[SII]6716/[SII]6731 = 0.6. UV observations show a nuclear ionizing
flux of Ni = 3.8 photons/s/cm2 at the Earth.
Assume the ionized gas has a single temperature and density, and fills
the aperture as a spherical region.
flux of 1.0×10-14 erg s-1 cm-2. The [OIII]5007
emission line has
a central wavelength of 5200A and a width (FWHM) of 10A. The
following flux ratios are measured : [OIII]5007/[OIII]4363 = 100 ;
[SII]6716/[SII]6731 = 0.6. UV observations show a nuclear ionizing
flux of Ni = 3.8 photons/s/cm2 at the Earth.
Assume the ionized gas has a single temperature and density, and fills
the aperture as a spherical region.
- What is the approximate electron density and temperature.
- What is the total volume and mass of ionized hydrogen.
- Use the redshift and aperture size to calculate the emission region
diameter (use Ho = 75 km s-1 Mpc-1) and hence the
filling factor for the ionized gas. Hopefully, you will find a small
filling factor and hence confirm the general view that the NLR is cloudy.
- We can estimate the number of clouds if we know the size of each
cloud, which we can estimate assuming the clouds are ionized just down
to their Stromgren depth (a somewhat risky assumption). First evaluate the
number of ionizing photons
striking unit area of the cloud surface, assuming a typical cloud to be
at a distance of half the aperture radius from the nucleus. Next evaluate
the Stromgren depth, Sd, by equating this ionizing flux to the total
number of recombinations in a column of depth Sd
(ie Ni =
 B Ne Np Sd). Taking this depth
to be the diameter of a typical cloud calculate the volume
of one cloud, and hence the total number of clouds.
B Ne Np Sd). Taking this depth
to be the diameter of a typical cloud calculate the volume
of one cloud, and hence the total number of clouds.
- Assuming no clouds
overlap and all are at the same distance from the nucleus (half the aperture
radius), what is the covering factor that the clouds present to the
nuclear radiation field --- ie what fraction of the nuclear radiation do
they intercept.
- Assuming that the velocity field is basically gravitational in origin,
estimate the total gravitating mass within the region. Compare this with
an estimate of the Black Hole mass assuming it is radiating at 10% of
the Eddington luminosity (assume all ionizing photons have an energy
of a photon at the Lyman edge, and that this constitutes the entire
nuclear luminosity). Is it likely that NLR kinematics are influenced
by the mass of the central black hole ?
(2) Anisotropic emission in AGN
Continuing with the path from observations to inferred properties, let's look
at evidence for anisotropic nuclear radiation field. The method makes use of extended
emission line regions. These off-nuclear regions have a different sight
line down to the nucleus that our own sight line. Spectra
from the off-nuclear regions allow us to calculate the nuclear luminosity
emerging in their direction, and this is often found to be different from
the nuclear luminosity emerging in our own direction --- i.e. the nuclear
luminosity emerges anisotropically.
A typical example might be the following: An active galaxy at redshift 5000
km/s is observed using an aperture placed 10 arcseconds away from
the nucleus. The following line ratios are found: [SII]6716 / [SII]6731 = 0.9 ;
[OIII]5007 / H = 18.0 ; [OI]6300 / H
= 18.0 ; [OI]6300 / H = 0.30 ; [NII]6584 / H
= 0.30 ; [NII]6584 / H =
0.63 ; [OII]3727 / [OIII]5007 = 0.18. An aperture placed over the nucleus
shows a power law non-stellar continuum the form F
=
0.63 ; [OII]3727 / [OIII]5007 = 0.18. An aperture placed over the nucleus
shows a power law non-stellar continuum the form F

 -1.5
with F
-1.5
with F = 6.8×10-25 erg/s/cm2/Hz at 5000A.
= 6.8×10-25 erg/s/cm2/Hz at 5000A.
- Plot the off-nuclear line ratios on the diagnostic diagrams shown
here to show
that the regions are likely to be photoionized by the central source
(and are not star forming HII regions, for example). Hand in printed versions of these figures.
- Use these diagrams to estimate the radiation parameter for the
off-nuclear gas, assuming solar abundances.
- Use the [SII] line ratio to estimate the electron density, and the
redshift (Ho = 75) to estimate the distance from the off-nuclear
region to the nucleus. Hence, using the standard equation which defines
the radiation parameter (eg 15.6 in the notes), estimate the
nuclear ionizing photon rate, Qi (the answer will be in number of
ionizing photons per second).
This value is the one appropriate for a line of sight towards the
off-nuclear gas.
- Now we need to compare this with the ionizing photon rate we
infer from our line of sight. First convert F
 at 5000A
to L
at 5000A
to L at 5000A (ignore K corrections and use Ho=75, and
remember L
at 5000A (ignore K corrections and use Ho=75, and
remember L has units of erg/s/Hz) --- to do this, of course, you will
be assuming that the source radiates isotropically (ie you multiply by
4
has units of erg/s/Hz) --- to do this, of course, you will
be assuming that the source radiates isotropically (ie you multiply by
4 d2 --- precisely the assumption we will ultimately be testing).
Next, derive the constant in L
d2 --- precisely the assumption we will ultimately be testing).
Next, derive the constant in L = k
= k  -1.5 using L
-1.5 using L at
5000A (dont forget to convert 5000A to Hz !). Now derive the
ionizing photon rate by integrating L
at
5000A (dont forget to convert 5000A to Hz !). Now derive the
ionizing photon rate by integrating L / h
/ h from
the Lyman edge (912A in Hz) to
from
the Lyman edge (912A in Hz) to 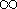 . This is the value appropriate
for our line of sight to the nucleus.
. This is the value appropriate
for our line of sight to the nucleus.
- Compare the ionizing luminosities estimated in `c' and `d' above
--- `d' should be about a factor 10 less (depending on exactly what
radiation parameter and electron dentity you estimated). Thus, the
off-nuclear ionized region is seeing a nuclear source which is about 10
times brighter than the one we see. Hence our conclusion that the
nuclear ionizing radiaton emerges anisotropically.
- A second test of this picture is to see if the blocked radiation
does ultimately emerge in our direction but in another waveband --- the IR,
for example, since our model places a dusty torus around the central
source. Continuing with our example : assume all radiation below 1 micron
is absorbed and re-radiated as a single black body of T = 50K,
what is the expected flux, in Jy, at 60 µm (one of the IRAS bands).




 flux of 1.0×10-14 erg s-1 cm-2. The [OIII]5007
emission line has
a central wavelength of 5200A and a width (FWHM) of 10A. The
following flux ratios are measured : [OIII]5007/[OIII]4363 = 100 ;
[SII]6716/[SII]6731 = 0.6. UV observations show a nuclear ionizing
flux of Ni = 3.8 photons/s/cm2 at the Earth.
Assume the ionized gas has a single temperature and density, and fills
the aperture as a spherical region.
flux of 1.0×10-14 erg s-1 cm-2. The [OIII]5007
emission line has
a central wavelength of 5200A and a width (FWHM) of 10A. The
following flux ratios are measured : [OIII]5007/[OIII]4363 = 100 ;
[SII]6716/[SII]6731 = 0.6. UV observations show a nuclear ionizing
flux of Ni = 3.8 photons/s/cm2 at the Earth.
Assume the ionized gas has a single temperature and density, and fills
the aperture as a spherical region.
 B Ne Np Sd). Taking this depth
to be the diameter of a typical cloud calculate the volume
of one cloud, and hence the total number of clouds.
B Ne Np Sd). Taking this depth
to be the diameter of a typical cloud calculate the volume
of one cloud, and hence the total number of clouds.
 = 18.0 ; [OI]6300 / H
= 18.0 ; [OI]6300 / H = 0.30 ; [NII]6584 / H
= 0.30 ; [NII]6584 / H =
0.63 ; [OII]3727 / [OIII]5007 = 0.18. An aperture placed over the nucleus
shows a power law non-stellar continuum the form F
=
0.63 ; [OII]3727 / [OIII]5007 = 0.18. An aperture placed over the nucleus
shows a power law non-stellar continuum the form F

 -1.5
with F
-1.5
with F = 6.8×10-25 erg/s/cm2/Hz at 5000A.
= 6.8×10-25 erg/s/cm2/Hz at 5000A.
 at 5000A
to L
at 5000A
to L at 5000A (ignore K corrections and use Ho=75, and
remember L
at 5000A (ignore K corrections and use Ho=75, and
remember L has units of erg/s/Hz) --- to do this, of course, you will
be assuming that the source radiates isotropically (ie you multiply by
4
has units of erg/s/Hz) --- to do this, of course, you will
be assuming that the source radiates isotropically (ie you multiply by
4 d2 --- precisely the assumption we will ultimately be testing).
Next, derive the constant in L
d2 --- precisely the assumption we will ultimately be testing).
Next, derive the constant in L = k
= k  -1.5 using L
-1.5 using L at
5000A (dont forget to convert 5000A to Hz !). Now derive the
ionizing photon rate by integrating L
at
5000A (dont forget to convert 5000A to Hz !). Now derive the
ionizing photon rate by integrating L / h
/ h from
the Lyman edge (912A in Hz) to
from
the Lyman edge (912A in Hz) to 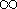 . This is the value appropriate
for our line of sight to the nucleus.
. This is the value appropriate
for our line of sight to the nucleus.