How can we measure the masses of systems as large as galaxies and galaxy clusters? Surely, we can neither count all their stars nor estimate their interstellar content very well. Galaxies are just too complex to take direct inventory of their material makeup. Instead, we must rely on indirect techniques. As usual, we turn to Newton's law of gravity.
Astronomers can calculate the masses of some spiral galaxies by determining their rotation curves, which plot rotation speed, obtained by measuring the Doppler shift of various spectral lines, versus distance from the galactic center. (Sec. 23.6) The mass within any given radius then follows directly from Newton's laws. Some rotation curves for nearby spirals are shown in Figure 24.19. They imply masses ranging from about 1011 to 5
1011 solar masses within about 25 kpc of the center—quite comparable to the results obtained for our own Galaxy using the same technique. Distant galaxies are generally too far away for such detailed curves to be drawn. Nevertheless, by observing the broadening of spectral lines—as discussed earlier in the context of the Tully—Fisher relation—we can still measure the overall rotation speed. Estimating the galaxy's size then leads to an estimate of its mass.
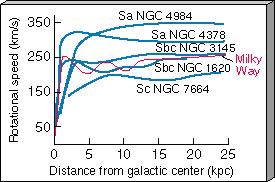
Figure 24.19 Rotation curves for some nearby spiral galaxies indicate masses of a few hundred billion times the mass of the Sun. The corresponding curve for our own Galaxy (Figure 23.19) is marked in red for comparison.
This approach is useful only for measuring the mass lying within about 50 kpc of a galaxy's center—the extent of the electromagnetic emission from stellar and interstellar material. To probe farther from the center, galactic astronomers turn to binary and multiple systems of galaxies, much as stellar astronomers study binary stars to determine stellar masses. If we knew the orbit size and period of a galaxy—galaxy binary system, the combined mass of the pair could be inferred from Kepler's third law, just as described in Chapter 17. (Sec. 17.9) However, there is a problem here. Unlike the stellar case, we cannot watch the galaxies travel even a small fraction of their entire orbit. Instead, the orbital period is usually simply estimated on the basis of two observations: (1) the velocities of the galaxies measured along our line of sight (Figure 24.20a) and (2) the present distance between the two galaxies. Masses obtained in this way, then, are fairly uncertain. However, we can combine many such measurements to obtain quite reliable statistical information about galaxy masses.
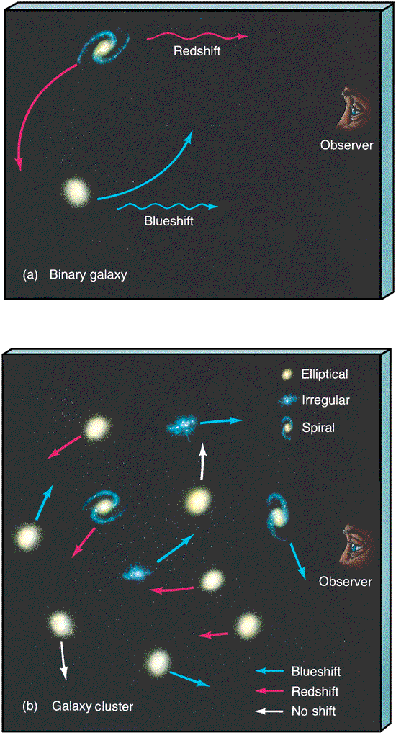
Figure 24.20 (a) In a binary galaxy system, galaxy masses may be estimated by observing the orbit of one galaxy about the other. (b) In a galaxy cluster, cluster masses are measured by observing the motion of many galaxies in the cluster and then estimating how much mass is needed to prevent the cluster from flying apart.
From investigations using these methods, we find that most normal spirals (the Milky Way Galaxy included) and large ellipticals contain between 1011 and 1012 solar masses of material. Irregular galaxies often contain less mass, about 108 to 1010 times that of the Sun. Dwarf ellipticals and irregulars can contain as little as 106 to 107 solar masses of material.
We can use another statistical technique to derive the combined mass of all the galaxies within a galaxy cluster. As depicted in Figure 24.20(b), each galaxy within a cluster moves relative to all other cluster members. As with binary galaxies, we cannot watch galaxies move around within a cluster—typical orbit periods are billions of years. But we can estimate the cluster's mass simply by determining, using Newtonian mechanics, how massive it must be in order to bind its galaxies gravitationally. Typical cluster masses obtained in this way lie in the range of 1013—1014 solar masses. Notice that this calculation gives us no information whatsoever about the masses of individual galaxies. It tells us only about the total mass of the entire cluster.
Radio observations indicate that the rotation curves of many spiral galaxies, such as those shown in Figure 24.19, remain flat (that is, do not decline and may even rise slightly) far beyond the visible image of the galaxy. We conclude that these spiral galaxies—and perhaps all other spiral galaxies as well—must have invisible dark halos similar to that surrounding the Milky Way Galaxy.
(Sec. 23.6) Depending on how far out these halos extend (and observations appear to indicate that they reach at least as far as 50 kpc from the center of a Milky Way—sized galaxy), spiral galaxies may contain 3 to 10 times more mass than can be accounted for in the form of visible matter. Some studies of elliptical galaxies suggest similarly large dark halos surrounding them too, but these observations are still far from conclusive.
Astronomers find an even greater discrepancy when they study galaxy clusters. Calculated cluster masses range from 10 to nearly 100 times the mass suggested by the light emitted by individual cluster galaxies. Stated another way, a lot more mass than we can see is needed to bind galaxy clusters. The problem of dark matter exists, then, not just in our own Milky Way Galaxy but also in other galaxies and, to an even greater degree, in galaxy clusters as well. It most likely applies to the entire universe. In that case, we must accept the fact that upward of 90 percent of the universe is composed of dark matter. As noted in Chapter 23, this matter is not just dark in the visible portion of the spectrum—it is invisible at all electromagnetic wavelengths.
As discussed in Chapter 23, many possible explanations for the dark matter have been suggested, ranging from stellar remnants of various sorts to exotic subatomic particles. (Sec. 23.6) Whatever its nature, however, the dark matter in clusters apparently cannot be simply the accumulation of smaller amounts of dark matter within individual galaxies. Even including the galaxies' dark halos, we still cannot account for all the dark matter in galaxy clusters. As we look on larger and larger scales, we find that a larger and larger fraction of the matter in the universe is dark.
Could the additional dark matter be diffuse intergalactic matter existing among the galaxies within the clusters—that is, intracluster gas? Until the late 1970s, astronomers had no observational evidence for intergalactic matter, either inside or outside galaxy clusters. Then satellites orbiting above Earth's atmosphere detected substantial amounts of X-ray radiation in the direction of many galaxy clusters. Figure 24.21 shows false-color X-ray images of two such clusters. The X-ray-emitting region is centered on, and comparable in size to, the visible image of the cluster. These X-ray observations demonstrated for the first time the existence of large amounts of invisible hot gas—at more than 10 million K—within galaxy clusters.
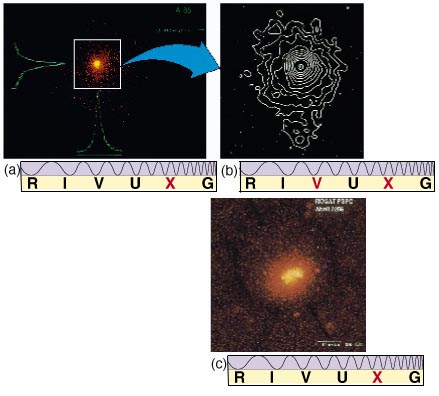
Figure 24.21 (a) X-ray image of Abell 85, an old, distant cluster of galaxies, taken by the Einstein X-ray satellite observatory. The cluster's X-ray emission is shown in orange. The green graphs display a smooth, peaked intensity profile centered on the cluster but not associated with individual galaxies. (b) The contour map of X-rays is superimposed on an optical photo, showing its X-rays peaked on Abell 85's central supergiant galaxy. Images like these demonstrated for the first time that the space among the galaxies within galaxy clusters is filled with superheated gas. (c) A ROSAT X-ray image of hot gas within another cluster of galaxies (called Abell 2256). The cluster is nearly a billion parsecs from Earth and measures about 3 Mpc in diameter.
How much matter have the X-ray satellites found? The observations suggest that at least as much matter—and in a few cases substantially more—exists within clusters in the form of hot gas as is visible in the form of stars. This is a lot of material, but it still doesn't solve the dark-matter problem. To account for the total masses of galaxy clusters implied by dynamical studies, we would have to find from 10 to 100 times more mass in gas than in stars. In the mid-1990s, ROSAT observations of gas within the Virgo cluster of galaxies actually compounded the problem (Figure 24.22). The gas was so hot that the amount of dark matter needed to bind it to the cluster and prevent it from dispersing into intercluster space was far greater than had previously been suspected to exist!
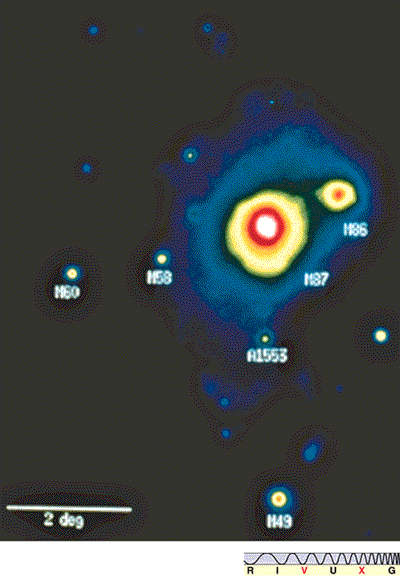
Figure 24.22 This combined optical and X-ray (ROSAT) image shows hot (107 K) X-ray-emitting gas (false-colored blue, increasing to yellow and red) within the rich Virgo Cluster of galaxies (see also Figure 24.15). The amount of mass required to bind this gas (especially that surrounding M87 shown in blue) to the cluster greatly exceeds the total mass of the galaxies themselves.
Astronomers have no evidence for gas beyond the well-defined galaxy clusters. "Extracluster" space is apparently empty of luminous material. Evidently, when the clusters formed eons ago, they did so very efficiently, sweeping up all the matter within any given region of the universe.