At present the universe is expanding. Will that expansion continue forever? And if not, what will happen next, and when? These are absolutely fundamental questions concerning the fate of the entire universe. Yet, remarkably, now that we know we can confidently apply our familiar Newtonian concepts of motion under gravity to the behavior of the universe, we can address these issues by considering a simpler and much more familiar problem.
Consider a rocket ship launched from the surface of a planet. What is the likely outcome of that motion? There are basically two possibilities, depending on the speed of the ship. If the launch speed is high enough, it will exceed the planet's escape speed, and the ship will never return to the surface.
(Sec. 25.5)
The speed will diminish because of the planet's gravitational pull, but it will never reach zero. The spacecraft leaves the planet on an unbound trajectory, as illustrated in Figure 26.8(a). Alternatively, if the ship's launch speed is lower than the escape speed, it will reach a maximum distance from the planet, then fall back to the surface. Its bound trajectory is shown in Figure 26.8(b).
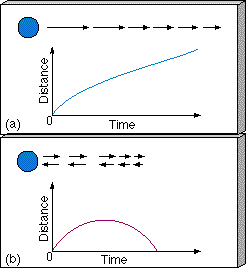
Figure 26.8 (a) A spacecraft (arrow) leaving a planet (blue ball) with a speed greater than the escape velocity leaves on an unbound trajectory (top). The graph (bottom) shows the distance between the ship and the planet as a function of time. (b) If the launch speed is less than the escape velocity, the ship eventually drops back to the planet. Its distance from the planet first rises, then falls.
Similar reasoning applies to the expansion of the universe. Reconsider Figure 26.7, but now imagine that A and B are galaxies at some known distance from each other, with their present relative velocity given by Hubble's law. The same two basic possibilities exist for these galaxies as for our spacecraft—the distance between them can increase forever, or it can increase for a while and then start to decrease. What's more, the cosmological principle says that, whatever the outcome, it must be the same for any two galaxies—in other words, the same statement applies to the universe as a whole. Thus, as illustrated in Figure 26.9, the universe has only two options: it can continue to expand forever—an unbound universe—or the present expansion will someday stop and turn around into a contraction—a bound universe.

Figure 26.9 Distance between two galaxies as a function of time in each of the three possible universes discussed in the text: unbound, bound, and marginally bound. The point where the three curves touch represents the present time.
The middle curve on Figure 26.9 marks the dividing line between these two possibilities. It shows a marginally bound universe that expands forever, but at an ever-decreasing rate, analogous to our rocket ship's leaving the planet with precisely the escape speed. The three curves are drawn so that they all pass through the same point at the present time. All are possible descriptions of the universe given its present size and expansion rate.
What determines which of these possibilities will actually occur? The answer is the density of the universe. In all cases gravity decelerates the expansion over time. The more matter there is—the denser the universe—the more "pull" there is against the expansion, just as the more mass a planet has, the less likely it is that a rocket ship can escape. In a high-density universe, there is enough mass to stop the expansion and cause a recollapse—the universe is bound. A low-density universe, conversely, is unbound and will expand forever. The dividing line between these two outcomes, the density corresponding to a marginally bound universe, is called the critical density. Its value depends on Hubble's constant—more matter is required to bind a more rapidly expanding universe. For H0 = 65 km/s/Mpc, the present critical density turns out to be 8 × 10-27 kg/m3 (which we will round off to 10-26 kg/m3). That's an extraordinarily low density—about five hydrogen atoms per cubic meter, a volume the size of a typical household closet. In more "cosmological" terms, it corresponds to about one Milky Way Galaxy (excluding the dark matter) per cubic megaparsec.
If the universe emerged from the Big Bang with a density above the critical value, then it contains enough matter to halt its own expansion, and the recession of the galaxies will eventually stop. At some time in the future, astronomers everywhere—on any planet within any galaxy—will announce that the radiation received from nearby galaxies is no longer redshifted. (The light from distant galaxies will still be redshifted, however, because we will see them as they were in the past, at a time when the universe was still expanding.) The bulk motion of the universe, and of the galaxies within, will be stilled—at least momentarily.
The expansion may stop, but the pull of gravity will not. The universe will begin to contract. Nearby galaxies will begin to show blueshifts, and both the density and the temperature of the universe will start to rise as matter collapses back onto itself. As illustrated in Figure 26.10(a), the universe will recollapse to a point, requiring just as much time to fall back as it took to rise. First galaxies, then stars will collide with increasing frequency and violence as the available space diminishes and the entire universe shrinks toward a superdense, superhot singularity much like the one from which it originated. The cosmos will ultimately experience a "heat death," in which all matter and life are destined to be incinerated. Some astronomers call the final collapse of this high-density universe the "Big Crunch."

Figure 26.10 (a) A high-density universe has a beginning, an end, and a finite lifetime. The lower frames illustrate its evolution, from explosion to maximum size to recollapse. (b) An oscillating universe has neither a beginning nor an end. Each expansion—contraction phase ends in a "bounce" that becomes the "Big Bang" of the next expansion. There is currently no information on whether this can actually occur. (c) A low-density universe expands forever from its explosive beginning. The upper curve represents a universe with density less than the critical value. The lower curve represents a universe with density exactly equal to the critical value.
Cosmologists do not know what will happen to the universe if it ever reaches the point of collapse. We cannot penetrate forward in time beyond the singularity at the Big Crunch any more than we can probe backward past the Big Bang—the laws of physics as we presently understand them are simply inadequate to describe these extreme conditions. However, some theorists speculate that with both density and temperature increasing as the contraction nears completion, the pressure may somehow be sufficient to overcome gravity, pushing the universe back out into another cycle of expansion. As depicted in Figure 26.10(b), the universe may not simply end—instead it may "bounce." A hypothetical universe having many—perhaps infinitely many—cycles of expansion and contraction could be the result. Bear in mind, though, that any discussion of the universe outside of the current cycle is pure speculation.
A quite different fate awaits the universe if its density is below the critical value. In that case its density always has been, and always will be, too small for gravity to cause it to recontract. As illustrated in Figure 26.10(c), such a low-density universe will expand forever. In this scenario the galaxies will continue to recede forever, their radiation weakening with increasing distance. In time an observer on Earth will see no galaxies in the sky beyond the Local Group (which is not itself expanding), even with the most powerful telescope. The rest of the observable universe will appear dark, the distant galaxies too faint to be seen. Eventually, the Milky Way and the Local Group too will peter out as their fuel supply is consumed. This universe will ultimately experience a "cold death." All radiation, matter, and life are eventually destined to freeze.
In the intermediate, critical-density case the universe contains just enough matter eventually to halt the expansion—but only after an infinitely long time. This universe will also expand forever, as indicated in Figure 26.10(c).