Whittle : EXTRAGALACTIC ASTRONOMY

 
     
 

1 : Preliminaries	6 :   Dynamics I	11 : Star Formation	16 : Cosmology
2 : Morphology	7 :   Ellipticals	12 : Interactions	17 : Structure Growth
3 : Surveys	8 :   Dynamics II	13 : Groups & Clusters	18 : Galaxy Formation
4 : Lum. Functions	9 :   Gas & Dust	14 : Nuclei & BHs	19 : Reionization & IGM
5 : Spirals	10 : Populations	15 : AGNs & Quasars	20 : Dark Matter

(1) Introduction

For reasons not yet fully understood, matter in the universe is organized into three basic structures:

• Atoms
• Stars
• Galaxies     -- the subject of this course

• ~1750 - 1850 :   recognition of basic existance
• ~1850 - 1930 :   recognition of basic properties
• ~1930 - present :   deeper understanding (structure, creation, evolution, sociality)

• atoms & stars :   understanding now mature
• galaxies :   becoming mature; now 30 yrs is a golden time.

    currently fertile area (astronomy is prominent amongst physical sciences)
    your careers will witness significant growth
    these notes will be of limited use when you teach the course, in ~20 yrs.

Let's first look briefly at some historical hightlights.

(2) Discovering Galaxies : Ours & Others

(a) Early Aims

• Early thinking (before 1923) focussed on two main questions :
  • What is the Milky Way   (Latin : Via Lactea)
    • initially : what is its shape and where is the sun
    • later : what is its size and internal motion

  • What are the Nebulae   (Latin : clouds)
    • initially : use "large" telescopes to find, catalog, and describe them
    • later : are they unresolved star groups, or genuinely nebulous (gaseous)
    • finally : are they internal to the MW, or external "island universes"

• As telescope apertures increased, the methods developed :
  • Visual photographic visual spectra photographic spectra

• The path of discovery was NOT linear, with discussion often polarized and ambiguous.

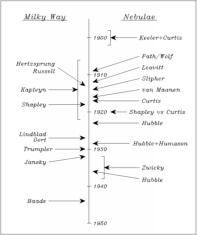
• Here are some simple time-line sketches identifying the key people/work

  1750 - 1900      [ figure ] 1900 - 1950      [ figure ] 1950 - present  [ figure ] all combined:    [ figure ]

(b) Before 1850 : Search & Discovery

• 1610 :   Galileo Galilei (Italian) uses early telescopes
  He realizes the Milky Way is composed of many stars [image]

• 1750 :   Thomas Wright (English) : [image]
  Publishes "An Original Theory of the Universe" in 1750
  giant spherical shell; we see tangent plane; God @ center
  stars orbit around, preventing them falling onto God
  

• 1755 :   Immanuel Kant (German) : [image]
  writes : "General Natural History & Theory of the Heavens"
  (1) rejects spherical shell
  (2) MW like huge solar system, rotating; origin from rotating cloud.
  (3) stars far from plane on different orbits
  (4) disks (like MW) project to ellipses
  (5) oval nebulae (seen by de Maupertius) = "Island Universe"
    remarkably precient, but not widely accepted through 1800s

• 1780s :   William Herschel (English) : [image]
  star counts MW = flat disk with sun @ center
  no size estimate
  ultimately recognizes wrong assumptions & retracts

• 1781 :   Charles Messier (French) : [image]
  completes first catalog of nebulae (109)

• 1770 - 1810 :   William & Caroline & John Herschel (English) : [image]
  all-sky survey     2500 nebulae
  used 18" (20 foot) reflecting telescope, diurnal sweeps
  extended to southern skies by son John at Cape of Good Hope (1834-9)
  some resolve into stars (clusters) others dont (gas?)
  speculation : uniform distribution of stars will gravitationally cluster
  

• 1845 :   William Parsons (3^rd Earl of Rosse) : [image]
  English Lord resident in central Ireland : Birr Castle
  36" then 72" (Leviathan of Parsonstown; largest until 100" Mt Wilson, 1917)
  spiral structure (eg M51, M33, M101)
  some have stars and gas (eg M42)     supports Kant's rotation idea
  1840s potato famine stops work; never achieves its potential

• 1864-68 :   William Huggins (English) : [image]
  telescopic visual spectra of nebulae
  1/3 emission lines (gaseous); 2/3 continuous (stellar)

• 1888 :   John Dreyer (Danish) [image] working at Birr Castle, compiles
  New General Catalog (NGC) :   7840 nebulae
  Index Catalog (IC) :   5086 more

• 1900s :   James Keeler and Herber Curtis (USA, Lick) use photography
  36" Crossley reflector @ Lick : [image]
  estimates ~120,000 nebulae accessible; ~50% are spiral

• 1906-22 :   Jacobus Kapteyn (Dutch) : detailed study of MW : [image]
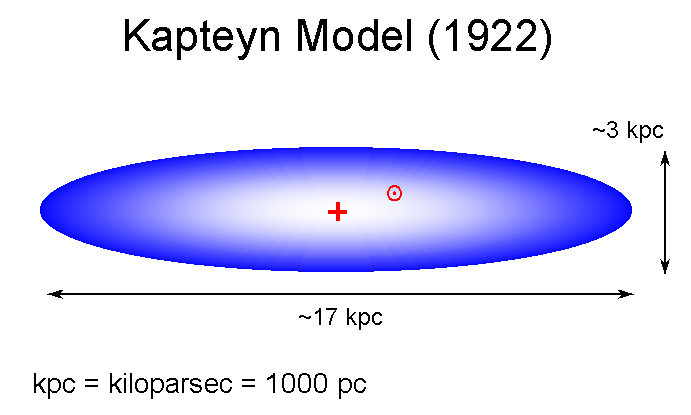
  surveys 200 areas :   star counts, proper motions, radial velocities
  concludes :   MW = thick disk, 5kpc radius, sun @ center
  considers absorption and finds some reddening, but
  assumes Rayleigh scattering so infers (wrongly) absorption unimportant

• 1912 :   Henrietta Leavitt (USA, Harvard) : [image]
  Period-Lumimosity relation for Cepheids in Magellanic Clouds
  tool for measuring distances

• 1914 :   Vesto Slipher (USA, Lowell) : [image]
  spectra of spirals (take 80 hours!)
  finds large velocities (eg M31 is -300 km/s)
  much larger than any MW stars

• 1918 :   Harlow Shapley (USA, Princeton/Harvard) :   [image]
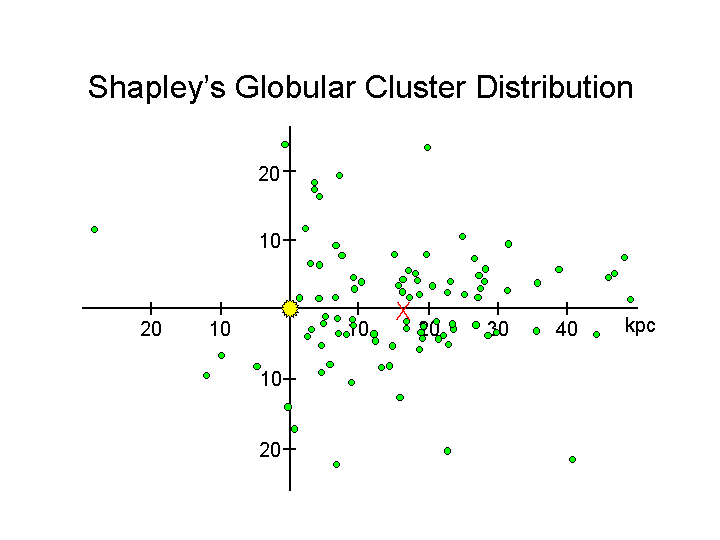
  uses Globular Clusters (GCs) to infer "Big Galaxy"
  diameter 100 kpc, sun ~15 kpc off-center
  10 x Kapteyn's galaxy; suggests absorption was the problem

• 1920 :   Shapley (no) - Curtis (yes) Debate :   "Are Spiral Nebulae Island Universes"   [image]
  public debate @ National Acadamy of Science, Washington DC.
  Short (30min) presentations, but summary articles published 1921
  surprisingly, Shapley "won" the debate, though Curtis was right.
  Shapley :
  new MW so big, inconceivable universe so much bigger
  van Maanen rotation rules out distant spirals
  Curtis :
  doubted Shapley's MW size
  range in size (0.01-2 deg) range in distance (1000x more than MW)
  Novae in M31 100 kpc away and size of Kapteyn's MW
  spectra show large doppler shifts, yet no proper motions
  some edge on spirals have dust lanes similar to MW (zone of avoidance) external

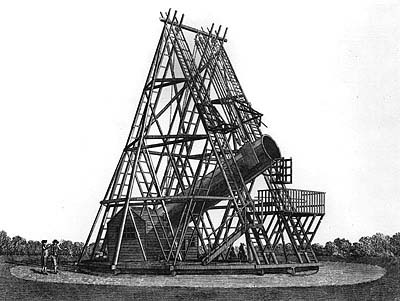
• 1923 :   Edwin Hubble (USA, Mt Wilson) :   uses the new 100" [image]
  finds Cepheids in M31 300 kpc (now, 770 kpc) external galaxy
  (centennial review of Hubble's career by Sandage : [ o-link ])

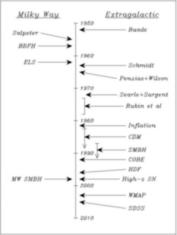
(d) 1925 - 1950 : Expanding Horizons

• 1927 :   Bertil Lindblad (Sweedish) and Jan Oort (Dutch) : [image]
  Lindblad predicts differential rotation near sun; Oort find it
  supports Shapley's MW with sun off-center (against Kapteyn's MW)
  however, derives smaller size than Shapley
  

• 1929 :   Edwin Hubble and Milton Humason (USA, Mt Wilson) [image]
  Finds redshift-distance relation (Hubble's Law)   (Original paper: [ o-link ])
  already expected from de Sitter's solutions to GR
  looked for by others; Hubble used distance ladder, including Cepheids
  1931 - includes many more galaxies
  H ~ 530 km/s/Mpc     2 Gyr age (less than earth !?)
  

• 1930 :   Robert Trumpler (USA, Lick) :
  compares sizes and CM diagrams of open clusters
  concludes absorption pervasive (~0.5mag/kpc, close to correct)
  nail in the coffin of Kapteyn's Milky Way

• 1936 :   Hubble : publishes galaxy classification (tuning fork) [image]
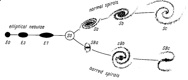
  uses names (early, late) influenced by Jean's theory of gravitational collapse
  eg E's = large gas cloud, evolves into spiral

• 1930s :   Fritz Zwicky (Swiss/USA, Cal Tech) : [image]
  measures galaxy velocities in Coma;
  infers dark matter needed if clusters are bound
  no one believes him

• 1944 :   Walter Baade (German/USA, Mt Wilson) : [image]
  observes Spiral bulges & Ellipticals (war time black-outs help)
  uncovers stellar populations :
  • Pop I :   blue supergiants in disks
  • Pop II :   red giants in bulges and Ellipticals

(e) 1950 - Present : Modern Developments

• 1952 :   Baade :   uses 200" to recalibrate Cepheid P-L relation
  depends on Pop I or II; previous work used wrong relation
    all distances doubled
    M31 is similar in size to MW
    Universe doubles in size (!)

• 1962 :   Eggen, Lynden-Bell & Sandage (ELS) : [image]
  Collapse model for formation of MW galaxy
  Accounts for position/kinematic/metallicity gradients
  Importance of ELS picture still debated

• 1963 :   Maartin Schmidt (German/USA, Cal Tech) : [image]
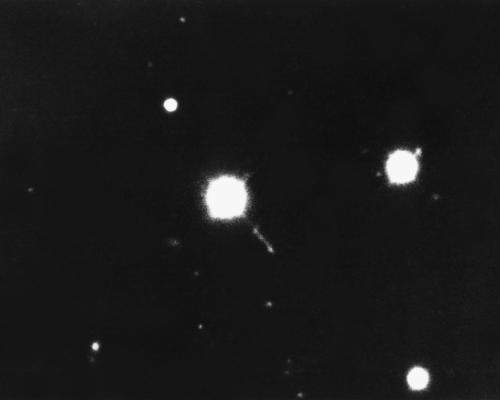
  Discovers Quasars (identifies redshift of 3C 273).

• 1965 :   Arno Penzias & Robert Wilson (USA, Bell Labs) : [image]
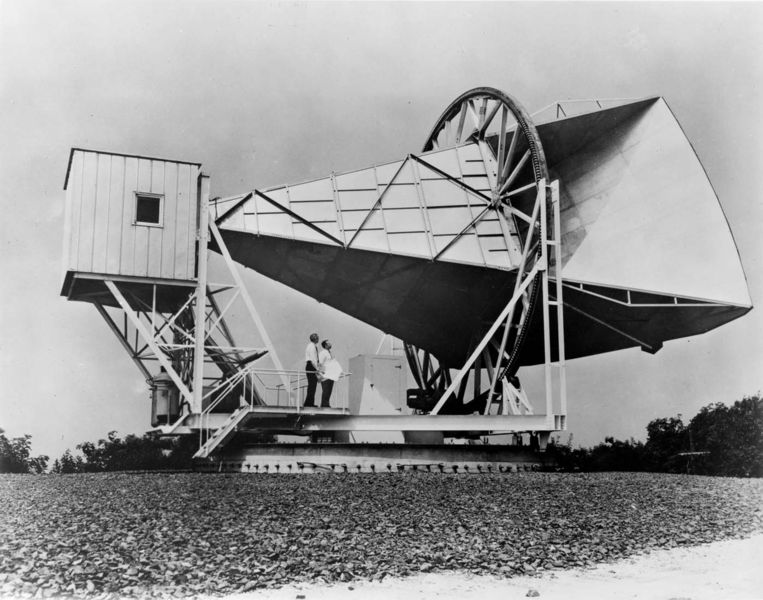
  Discover Cosmic Microwave Background (CMB)
  Strong support for Hot Big Bang model

• 1972 :   Leonard Searle & Wal Sargent (USA, Cal Tech) :
  measure 24% He basiline in low metallicity Dwarfs
  consistent with Big Bang nucleosynthesis

• 1970s :   Vera Rubin et al. (USA, Carnegie) : [image]
  infers dark matter from spiral rotation curves
  inspires Cold Dark Matter (CDM) models of 80s-90s

• 1978 :   Len Searle & Robert Zinn (USA, Cal Tech) :
  Abundance analysis of MW Globular Clusters: infer range of ages
  Suggest MW halo built up by accretion of fragments after main formation

• 1980 :   Alan Guth & Alexei Starobinski (USA; USSR) : [image]
  Independently conceive of early period of extremely rapid, accelerated expansion.
  Guth calls this "inflation": solves several deep problems.
  Provides natural explanation for creation of everything, and launching the expansion.

• 1992 :   COBE (NASA): [image]
  measures stunningly accurate black body spectrum
  finds slight (10^-5) anisotropies in CMB     pregalactic structure

• 1996 :   HST's HDF (NASA): [image]
  galaxies down to 29^m; out to z~3; total ~10¹⁰
  young galaxies visibly different
  early star formation rate is high ("Madau" plot)

• 1998 :   High-z SN Projects (USA, Berkeley & Harvard): [image]
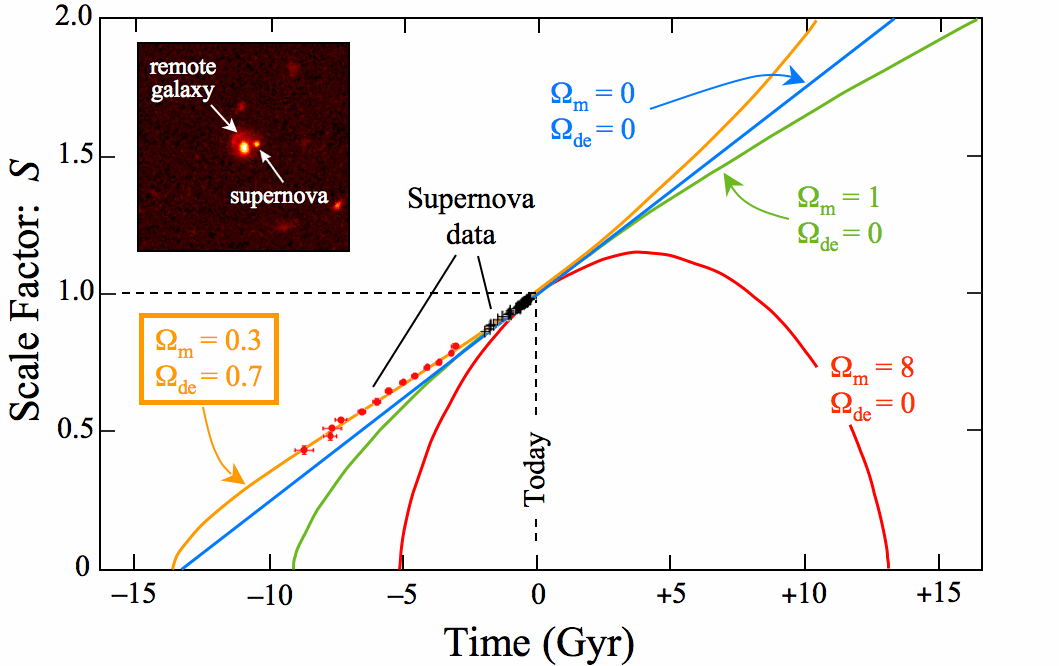
  Two groups use Type Ia SN as standard candles out to z ~ 1
  Both find evidence for non-zero cosmological constant (universe accelerating)

• 2003 :   2dF (& SDSS) Galaxy Surveys (UK/Australia & USA): [image]
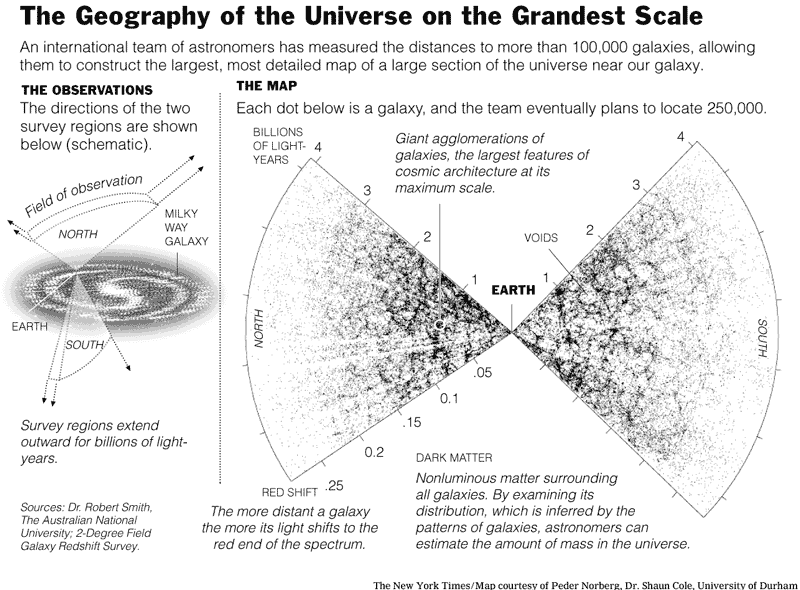
  The first of the large scale galaxy redshift surveys is completed (2dF)
  250,000 galaxy redshifts out to z~0.1 allow detailed analysis of large scale structure.
  SDSS completed later (800,000 galaxy redshifts) but with more detailed information.

• 2003 :   WMAP (NASA) : [image]
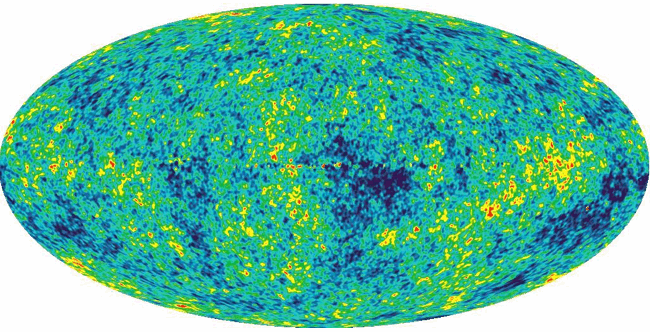
  CMB power spectrum measured; includes accoustic peaks 1,2,(~3)
  inspires concordance model with "high" accuracy (few %)
  combines : WMAP;   SN-1a;   2dFGRS;   HST-H_o;   BBNS; to find : [image]
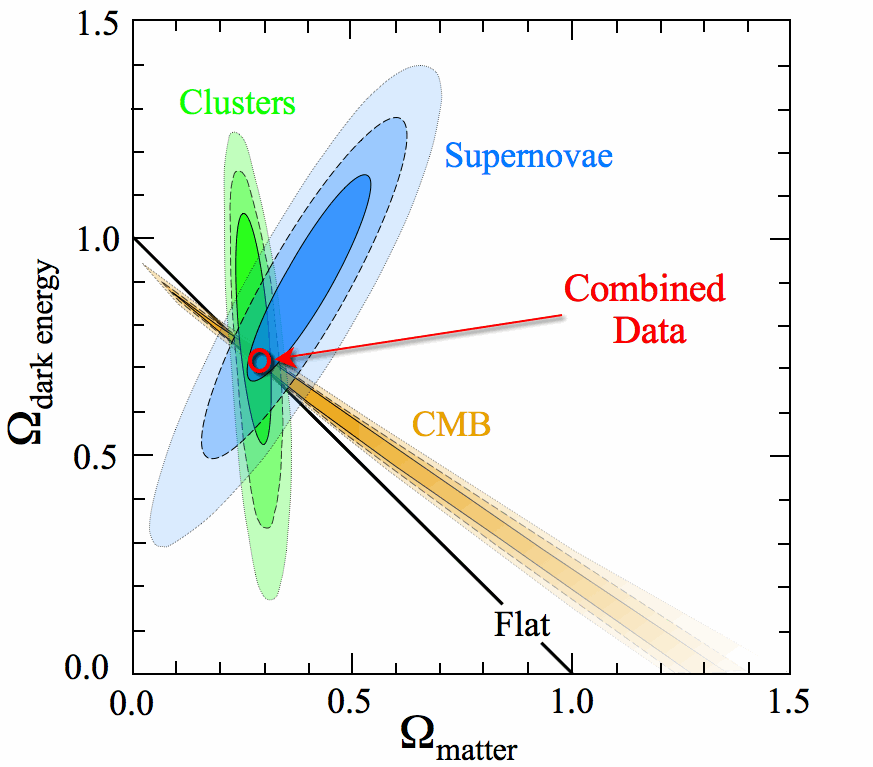
  • flat geometry
  • 70% Dark Energy; 26% Dark Matter; 4% Baryonic Matter
  • age 13.7 Gyr
  • initial fluctuation spectrum is power law, index -1 (consistent with inflation)

(3) Preliminaries

(a) Basic Scales

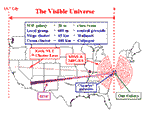
The following ASTRO-101 type diagrams remind us of the relative size of galaxies and our visible horizon

• Remind yourself, using simple scale models, just how BIG the Universe is:   [image].

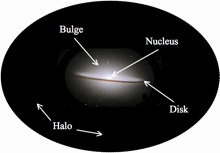
(b) Galaxies are Multicomponent Systems

• Three constituents, with rough mass ratio 1/10/100 : Gas / Stars / Dark Matter
  
  The first two have complex identity :
  • Gas:     different phases; dynamics; composition; (like "weather")
  • Stars:  different ages; locations; kinematics; metallicities; (like "cars")
  The third is simpler but more enigmatic:
  • DM:      collisionless "gas" (of WIMPs?); huge; ~smooth; centrally concentrated

• Several components, with varying prominence depending on galaxy type [image].
  • Nucleus: dense; star formation; supermassive black hole
  • Bulge:     spheroidal; mixed ages; kinematically "hot" & little rotation
  • Disk:       gas & stars; younger; star formation; spiral arms; kinematically "cold" & rotates
  • Halo:       low density; GCs present; old; Dark Matter dominates;

    Note :   Dark Matter dominates on large scales only
                 bulge & disk dynamics determined by stars & gas alone

(c) Colors and Spectra

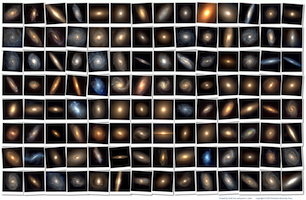
• A montage of SDSS galaxies shows a limited range of colors: blue -- red   [image]
  Statistical analysis suggests the color distribution is roughly bimodal   [image].
  Crudely speaking:
  blue = younger population
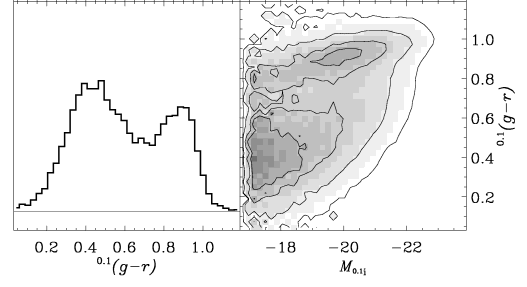
  red   = older population   (actually, more like yellow/orange)

• This can be understood in terms of stellar evolution:
  Following an episode of star formation, the main sequence "erodes" downwards. [image].
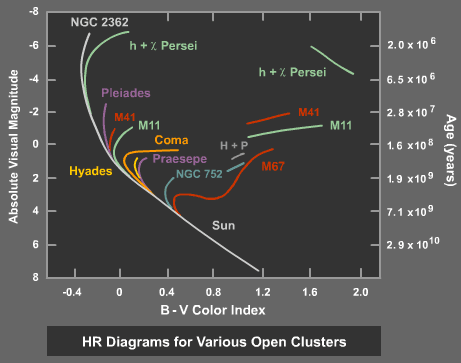
  Young population: light is dominated by higher mass main sequence stars.
  Older population: light is dominated by red giants.
  (In both cases these are a minor but luminous sub-population.)
  

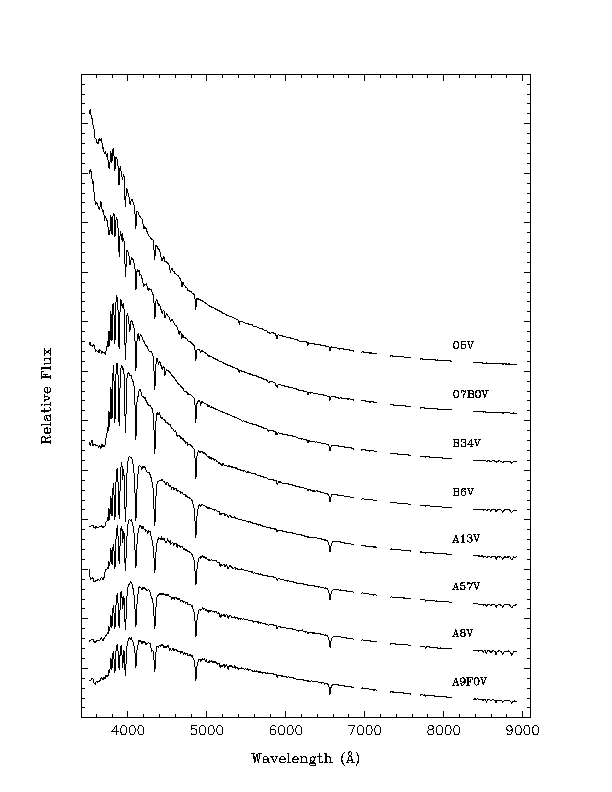
• Spectra show in more detail these population differences
  Star spectra primarily follow the spectral type [image].
  Galaxy spectra show mixed populations [image].
  

• Analysis of these spectra can reveal many properties:
  • population mix of stars
    
  • current star formation (e.g. emission lines)
    
  • metallicity (fraction of heavy elements, i.e. beyond He)
    
  • kinematics of gas and stars: rotation and dispersion.

(d) Useful Units

Calculations of galaxy properties are greatly simplified with sensible units (see also: Toolbox).
Rather than "mks" or "cgs" for length/mass/time, we can use:

"psm" :   parsec,   solar mass,   Megayear   :   pc, M, Myr

There are a number of nice features to this system:

1. (1) Velocity in psm units, pc/Myr, is the same as km/s (within 2%; 1pc/Myr = 0.9778 km/s)
   (recall the pnemonic: "a kilometer per second is a parsec in a million years ")
2. (2) Newton's constant: G = 4.50 × 10^-3   (4.49846 × 10^-3)
   its units are:   (pc³/M) Myr^{-2     -1} Myr^-2       (km/s)² pc M^-1
3. (3) Equations, such as   M = R V² / G,   directly accept and yield observational values
4. (4) Densities, _psm, are in M/pc^-3 = 6.76 × 10^-23 gm cm^-3 = 40.4 m_p cm^-3 = 3.60 × 10⁶ h²_crit
5. (5) Frequencies, Myr^-1, are also velocity gradients: km/s/pc
6. (6) Crossing/collapse times: R(pc) / V(km/s) = 1 / (G )^½   are in Myr.
   

Some examples illustrate psm units, and introduce basic galaxy properties :
(see homework for further examples).

• Estimate the mass interior to the sun's orbit (R~8kpc; V~220 km/s)
  use (3): M ~ RV² / G     8000 x 220² / 4.5 x 10^-3 ~ 8.6 x 10¹⁰ M

• Whats the density at the galactic center, where V ~100 km/s @ R ~1pc?
  use (6) : R²/V² = 1 / (G )
    = V²/(G R²)   = 100² / (4.5x10^-3 x 1²)   =   2.2 x 10⁶ M/pc^-3

• What's the Schwarzchild radius of a 10⁸ M black hole ?
  use R_s = 2GM/c² = 2 × 4.5 × 10^-3 × 10⁸ / (3 × 10⁵)² = 1.0 × 10^-5 pc = 2 AU

• What's the Hubble time for H_o = 75 km/s/Mpc ?
  use (5) : t_H(Myr) = 1 / (75 km/s/Mpc) = 1 Mpc / (75 km/s)
  = 10⁶ / 75 = 1.33 × 10⁴ Myr = 13.3 Gyr

There are a few extensions to the psm system which can, at times, be useful:

7. psm energy units: peu (Mpc²Myr^-2) = 1.89 × 10³⁶ Joules
8. psm luminosity units: plu (peu/Myr) = 5.97 × 10²² Watt = 1.56 × 10^-4 L
9. mass/luminosity units: M/plu = 3.33 × 10⁷ kg/Watt = 6400 (M/L)
10. linear momentum: pmu (Mpc/Myr Mkm/s) = 1.85 × 10³³ kg m s^-1  
11. angular momentum: pamu (Mpc²Myr^-1 M km/s pc) = 5.71 × 10⁴⁹ kg m² s^-1
12. force (momentum flux): pfu (Mpc/Myr² Mkm/s/Myr) = 5.86 × 10¹⁹ N
13. acceleration: pau (pc/Myr² km/s Myr^-1) = 3.09 × 10^-11 m s^-2

A few more examples help illustrate:

• What's the gravitational luminosity of a galaxy merger (M ~ 10¹¹M in R ~ 10 kpc)
  Use (6) for collapse time ~ (G)^-½ ~ (4.5 × 10^-3× 10¹¹/20000³)^-½ ~ 130 Myr
  Energy of collapse ~ GM²/R ~ 4.5 × 10¹⁵ peu
  Gravitational luminosity = 3.5 × 10¹³ plu = 5.4 × 10⁹ L
  (much less than ~10¹¹ L from typical star formation).

• What's the ejection velocity of a 10 M supernova envelope of energy 10⁴⁶ J ?
  Energy is ~5 × 10⁹ peu ~ ½MV² V ~ 32,000 km/s

• What's the mechanical luminosity and force of a 1000 km/s AGN jet carrying 10 M yr^-1 ?
  L = ½ Mdot V² = ½ × 10⁷ × 10⁶ = 5 × 10¹²   plu  =  3 × 10⁴² erg s^-1
  F = Mdot V = 10⁷ × 10³ = 10¹⁰ pfu  =  5.9 × 10³⁴ dyne

(e) Magnitude Systems and Surface Brightness

The previous section deals only with dynamical variables : V, R, t, M.
Let's introduce starlight into the mix, not least because it is easy to measure.

Astronomers use two systems: magnitudes and fluxes (each with apparent and intrinsic)
it can sometimes be tricky jumping back and forth between these systems.

• Magnitudes: 
  The generic magnitude is defined:

  m  =  const  -2.5 log10[flux]  =  -2.5 log10[flux / flux_0]

  1.1

  
  where flux_0 is a reference flux for a star with m = 0.0 (usually Vega), and is filter-specific.
  Typically, m ~ 12 - 14 (nearby galaxies); 16 - 18 (distant galaxies); 21 - 25 (very distant galaxies).
  Apparent magnitudes, m, include information on both intrinsic luminosity and distance.

  Absolute magnitude (M) is defined as the apparent magnitude (m) were the object at 10pc, i.e.:
  

  m = const - 2.5 log f_{d pc}
  M = const - 2.5 log f_10pc

  but from the inverse square law:

  f_10pc = f_{d pc} × (d_pc / 10)²

  so, substituting:

  M = const - 2.5 log [f_{d pc} × (d_pc / 10)²]
  M = const - 2.5 log f_{d pc} - 2.5 log d_pc² - 2.5 log (1/100)

  Giving the well-known relation:

  M  =  m  -  5 log dpc  +  5

  1.2

  
  
  By placing everything at 10pc, absolute magnitudes are related to an object's luminosity
  M ~ -10 to -17 (dwarfs);
  M ~ -18 to -21 (normal galaxies);
  M ~ -22 to -24 (giant galaxies & QSOs).

• Solar magnitudes and fluxes: 
  Often, we express luminosities relative to the sun (e.g. 3 × 10⁸ L_V,)
  The sun's absolute magnitude in band X = U,B,V,R,I is M_X, = 5.66, 5.47, 4.82, 4.28, 3.94.
  Hence, an object with absolute magnitude M_X, has luminosity:

  LX  =  dex[ -0.4(MX - MX,) ]   LX,

  1.3

• Surface Brightness: 
  Extended objects have surface brightness, µ in mag arcsec^-2   (mag/ss; sometimes written )
  Since µ is independent of distance it immediately gives the surface luminosity density, I L pc^-2
  e.g. using M_,B from above, we find (see homework) :

  µB  =  27.04  -  2.5 log(IB)

  1.4

  
  
  and in general, for U,B,V,R,I, the constant is: 27.23, 27.04, 26.39, 25.85, 25.51.

• Example: 
  M87 has a central surface brightness µ_V = 17 mag/ss.
    the core has projected luminosity density: I_V = dex[ -0.4(17 - 26.39)]  =  5,700 L_V, pc^-2.
  if the core radius is 10 arcsec, what's the core's apparent magnitude?
    m_core  ~  17 - 2.5 log [×10²]  =  10.75
  for a distance of 15 Mpc, what's the total core luminosity?
    M = m - 5log d_pc + 5 = -20.13, giving L = dex[-0.4(-20.13 - 4.82)] = 9.55 × 10⁹ L_V,
  Using 10 arcsec = 10 × 15 × 10⁶/206265 = 730 pc, we find a luminosity density:
    j_core ~ I_core / 2 r_core ~ 3.9 L_V, pc^-3.
  to find the mass density requires a mass-to-light ratio, which is our next topic:

(f) Mass to Light Ratios

Light and dynamics are coupled using "Mass to Light Ratios (M/L)".
• "Mass to Light" (M/L) ratios are important for two reasons :
  • they allow us to estimate mass (important but difficult to measure)
    using light (easy to measure)

  • they tell us about the content of a system,
    eg (M/L) values differ :   pop I < pop II < galaxy+halo < clusters

• Solar units are used :   where (M/L)     M/L     1
  Physical units :   kg/Watt are not generally used
  [conversion: (M/L)_,bol = 5173 kg/Watt = 0.5173 gm/(erg/s) ]
  (M/L) is expressed at a given waveband, most commonly B,V,I,K, or bolometric (all ).
  e.g. for waveband "X", using absolute magnitudes :

  (M/L)X  =  M/M  /  LX/L,X  =  M/M  /  dex[ -0.4( MX  -  M,X) ]

  1.5

  
  where M_X & M_X are X-band absolute magnitudes of the object & sun;
  and L_X & L_X are X-band luminosities of the object & sun
  and X = U,B,V,R,I,K,bol   and   M_X = 5.66, 5.47, 4.82, 4.28, 3.94, 3.33, 4.74
  note : (M/L)_X is the same for all X only if the object and sun have the same colors
  (careful: M used for both mass and absolute magnitude here - sorry)

  One can also use luminosities (usually only bolometric)
  L_,bol = 3.84 x 10³³ erg s^-1   and M_bol = -2.5 log(L_bol / L_,bol) + 4.74

• For main sequence stars, we have L M^3.5, giving :   (M/L) M^-2.5 L^-0.71
  showing, as one expects, later spectral types have higher M/L.
  eg K stars : M ~ 0.5M     M/L ~ 10; A stars : M ~ 2.0M     M/L ~ 0.1

• For composite systems, M/L reflects the average M/L over the population
  • Pop I (young) :   massive stars dominate light; low mass stars dominate mass
    
  • Pop II (old) :   giants dominate light; M.S. stars dominate mass
    Typical galaxy (& solar neighborhood) has M/L_V ~ 6 , M/L_B ~ 10
    In general : M/L increases with age and metallicity
    Maximum range : 2 < M/L_B < 20.

  Dark components further increase these values, eg

  • SMBH in galaxy nuclei
  • Dark Matter in galaxy halos

  More specifically, for main sequence stars and composite systems in V :

  Type M / M MV LV / L,V (M/L)V O5 60 -5.7 16,140 0.0037 B5 5.9 -1.2 255 0.023 A5 2.0 +1.95 14 0.14 F5 1.4 +3.5 3.4 0.41 G5 0.92 +5.1 0.77 1.19 K5 0.67 +6.4 0.23 2.87 M5 0.21 +12.3 0.001 206

  System (M/L)V Reason HII region 0.3 - 1 Pop I only Spiral Disk 2 - 5 Pop I + II Bulges / Ellipticals 8 - 15 Pop II Nucleus (no AGN) 10 - 50 BH present Galaxy + halo 20 - 50 DM important Clusters 100 - 500 DM dominates Universe ~1000 DM dominates

(g) Cosmology 101

• The Hubble Law
  The most basic piece of cosmology is the Hubble Law, which arises from Cosmic expansion.
  
  v = H₀ × d  
  where v is recession velocity, d is distance, and H₀ is Hubble's constant ~ 72 kms/s/Mpc
  
  Example: what's the distance to a galaxy with z = 0.02?
  v cz = 6000 km/s,
  so d = v / H₀ = 6000 / 72 = 83.3 Mpc = 272 Mly
  This now allows you to calculate luminosities & linear sizes from fluxes & angular sizes.

  Note: at higher z (e.g. > 0.3), this equation won't work, and one needs a more sophisticated approach.
  Also, at very low z, peculiar velocities can be significant introduce errors to distances.

• Use of Scaled Hubble Constant: h
  For decades, H_o was uncertain to ~50%
  It was/is useful, therefore, to set H_o to 100h km/s/Mpc with h kept explicit
  h appears once for each redshift-distance, with a power of opposite sign: e.g.
  
  The distance to Coma, cz ~ 6000 km/s, is 60h^-1 Mpc
  The luminosity of 3C 123 is 3x10⁴⁴h^-2 erg/s
  3C 123 has M_B ~ -24.5 + 5log(h)   [recall m - M = 5log(d) - 5]
  The jet in 3C 123 has length 150h^-1 kpc   [length = angle x distance]
  The core mass of NGC 1234 is 2x10¹⁰h^-1 M   [M ~ RV²/G, & R d]
  Its luminosity density is 1.6h L pc^-3   [h^-2 / h^-3]
  Its M/L ratio is 10h solar units   [h^-1 / h^-2]

  Note that h does not appear for non-redshift distances (eg Cepheid distances).
  Although we now know h=0.72 (with ~5% uncertainty), its good to keep using it.

• Concordance Model Parameters
  After WMAP, the various cosmological datasets have yielded a robust cosmological model.
  The total density is equal to the critical density (_tot = 1.00) so the spatial geometry is Euclidean.
  The breakdown of today's densities is: _b = 0.04, _dm = 0.23, _de = 0.73, _r = 8.4 × 10^-5.
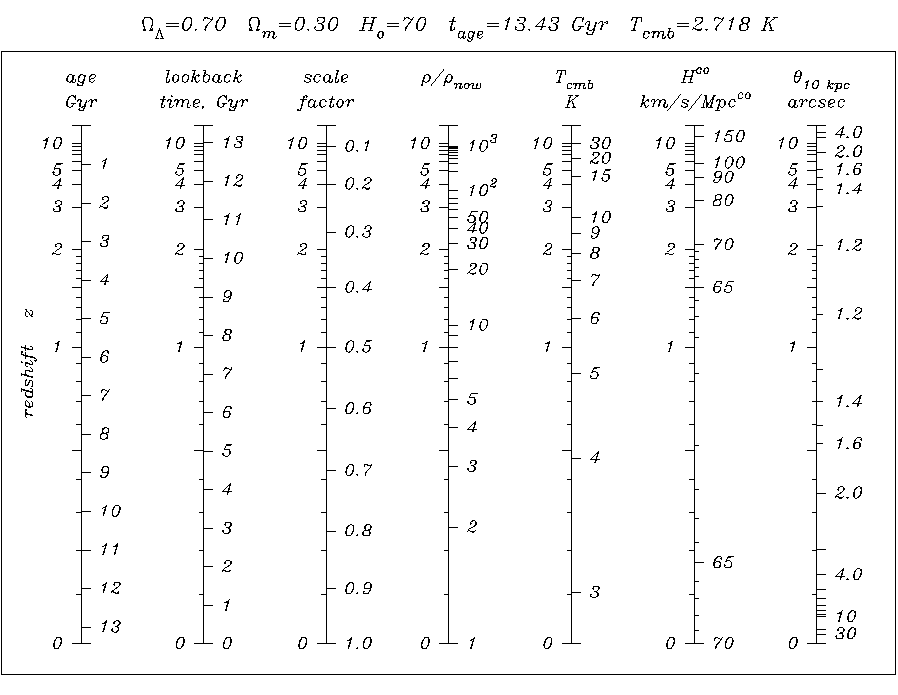  These are routinely used to define the relation between redshift and other important parameters, including cosmic time   [image]

• Intermediate & High Redshift
  It is now routine to ask how any properties change with redshift (i.e. cosmic epoch)
  It is therefore useful to have a basic feel for the link between z and lookback time.
  • z ~ 1 is ~60% lookback time (LBT), with cosmic age ~6 Gyr
  • coasting (changover from de to ac-celeration) occurs at z~0.65 or ~45% LBT
  • high-z galaxies and QSOs at z~4-6 are at ~90% LBT, age ~1Gyr
  • recombination is at z=1100, T=3300K, age=380 kyr, ~10³ cm^-3
  • at recombination, 10kpc subtends ~2.8 arcmin, or 1 deg ~170 kpc
  • matter/energy equality occurs at z~3300, T~10,000K, age~50 kyr.

This concludes our introduction to the subject of Extragalactic Astronomy
We are now ready to start, relatively gently, with Topic 2 : Galaxy Morphology.