Notice we have three related variables here: cosmic time, t; scale factor, a; and
redshift, z.
you can move between them with the following important relations between their differentials:
|
dt = tH,o da / aE(a) = -tH,o dz / (1+z)E(z) and da = -dz/(1+z)2 = -a2dz |
where tH,o = 1 / Ho is the Hubble time; one can also use the Hubble radius c dt = rH,o da / aE(a) etc.
For example, the relation for current cosmic age is simply:
Let's now look at the time evolution of some specific FRW world models.
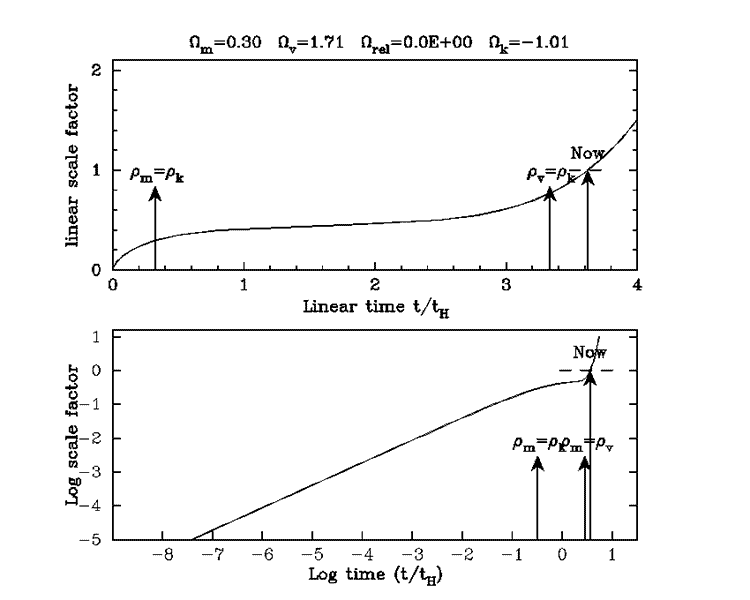
(ii) The Concordance Model
The real Universe has several components; with current densities:
 v,o = 0.73; v,o = 0.73;
 m,o = 0.27; m,o = 0.27;  r,o
= 8.4 × 10-5; r,o
= 8.4 × 10-5;  t,o = 1.00 ( t,o = 1.00 ( k,o = 1 - k,o = 1 -  t,o = 0) t,o = 0) |
Integrating the general relation gives a current age 0.988 tH,o and a(t)
curve shown here: [image]

In practice, each term is dominant over a certain range in a, giving three
eras :
during the radiation era: E(a) 
 r,o½ a-2
r,o½ a-2
during the matter era: E(a) 
 m,o½ a-3/2
m,o½ a-3/2
during the dark energy era: E(a) 
 v,o½
v,o½

Taken individually, these yield reasonable approximations for a(t) over most
of cosmic history.
This [ image ] shows the
approximations and their errors, while the table gives the functional forms.
[The Toolbox includes a more
extensive set, including Hubble radius, particle and event horizons.]
| Approximations to the Concordance model
|
Radiation era: E(a) 
 r,o½ a-2 r,o½ a-2 |
t = tH,o  da / a E(a) (0 to a) da / a E(a) (0 to a)  ½ tH,o ½ tH,o
 r,o-½ a2 r,o-½ a2  741 a2 h72-1 Gyr = 2.34 x 1019 a2 h72-1 sec 741 a2 h72-1 Gyr = 2.34 x 1019 a2 h72-1 sec
|
a  1.16 x 10-6 h72½ tyr½ 1.16 x 10-6 h72½ tyr½  2.09 x 10-10 h72½ ts½ 2.09 x 10-10 h72½ ts½
|
Matter era: E(a) 
 m,o½ a-3/2 m,o½ a-3/2 |
t = tH,o  da / a E(a) (0 to a) da / a E(a) (0 to a)  2/3 tH,o 2/3 tH,o
 m,o-½ a3/2 m,o-½ a3/2  17.4 a3/2 h72-1 Gyr 17.4 a3/2 h72-1 Gyr
|
a  1.49 x 10-7 h722/3 tyr2/3 1.49 x 10-7 h722/3 tyr2/3
|
Dark Energy Era: E(a) 
 v,o½ v,o½ |
t - tnow =  t = tH,o t = tH,o  da / a E(a) (1 to a) da / a E(a) (1 to a)  tH,o tH,o
 v,o-½ ln(a) v,o-½ ln(a)  15.9 ln(a) h72-1 Gyr 15.9 ln(a) h72-1 Gyr
|
a  exp[ exp[
 tGyr h72 / 15.9 ] (tnow = 0.988 tH,o) tGyr h72 / 15.9 ] (tnow = 0.988 tH,o)
|
Two details:
Formally, the exponential term has infinite past, so it is sensible
to integrate from a=1, the current time.
The matter solution is improved slightly by adding 39,000 years
to correct the integral over the radiation era.
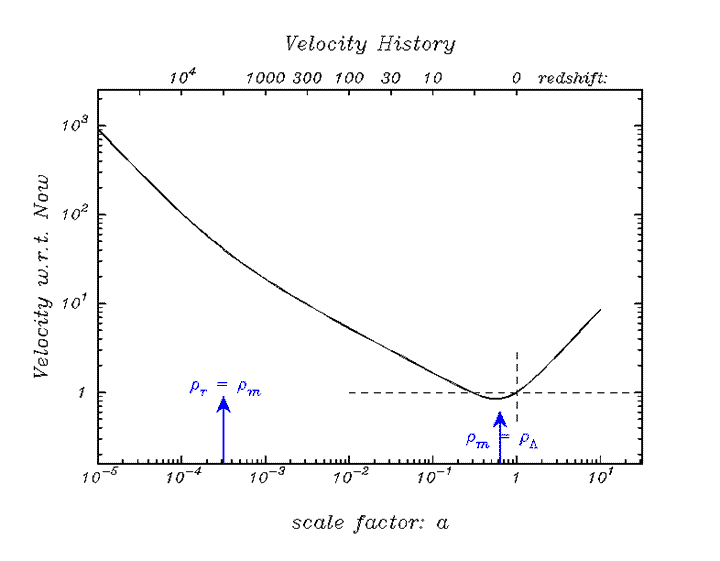
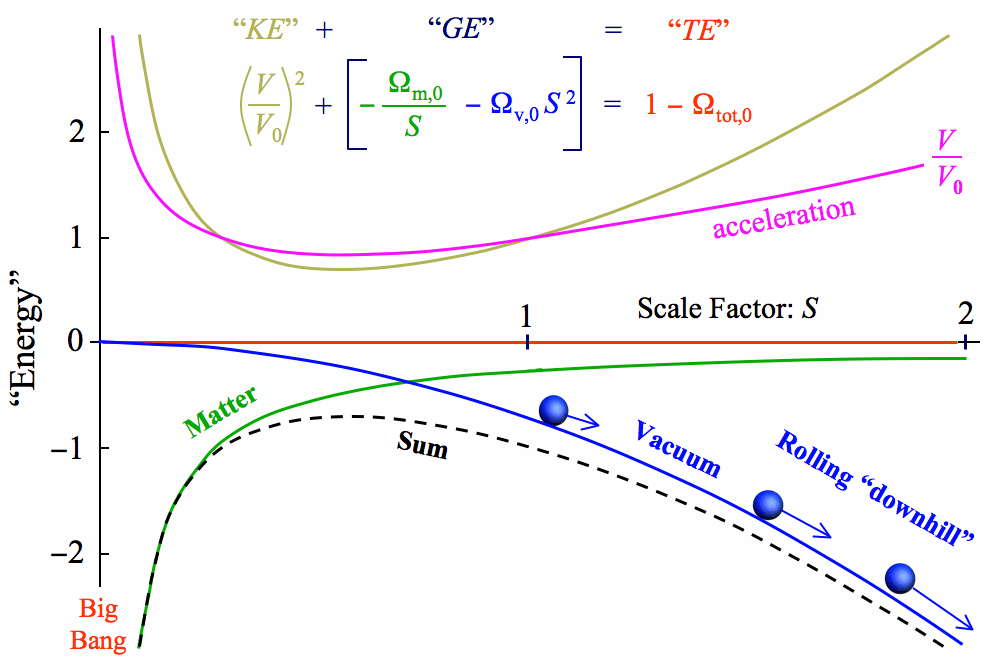
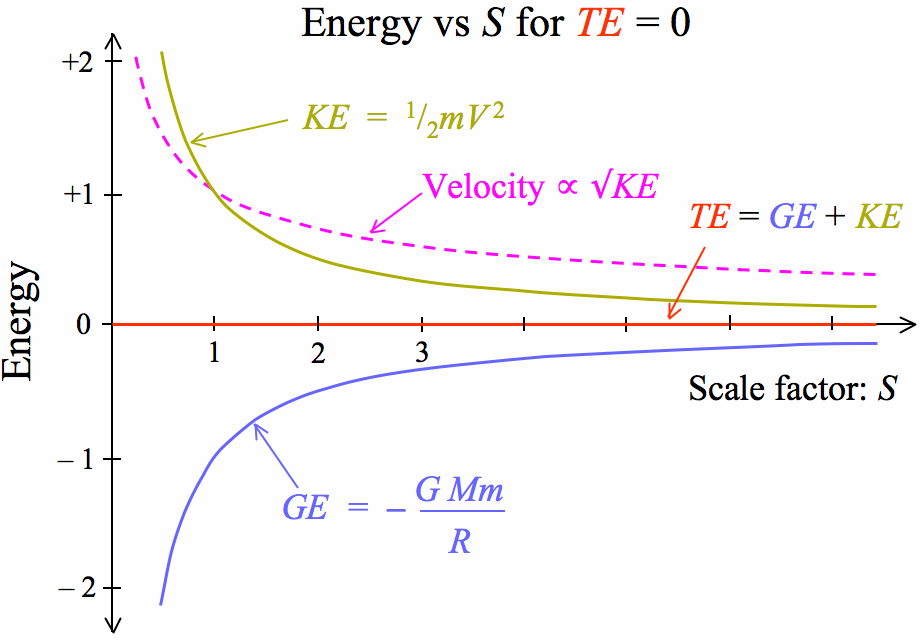
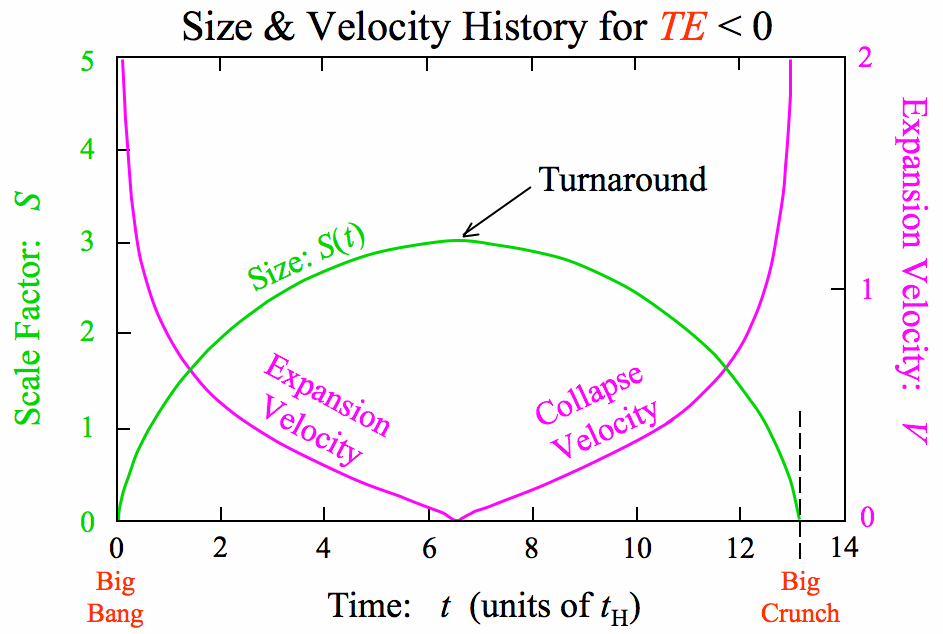
(iii) Velocity History and Newtonian Energy Diagrams
There is a remarkably transparent way to present the solutions to the Friemdann equations.
It mirrors the Newtonian analysis, using (dimensionless) kinetic, gravitational, and total energies.
Follow the above analysis, starting from the first line:
Introduce the velocity factor, v/vo = Hr / Horo = a H/Ho = (da/dt) / Ho
and express the time-varying density  t in terms of today's Omegas, we get:
t in terms of today's Omegas, we get:
(v/vo)2 + [ - m,o/a - m,o/a - r,o/a2 - r,o/a2 - v,oa2 ] = 1 - v,oa2 ] = 1 -  t,o t,o
"KE" "PE" "TE" (const)
|
Or using the previous notation: v/vo = a E(a).
Of course, to convert v(a) to a(t) we still need to integrate, just as before:
v/vo = (da/dt) / Ho = a E(a) giving:
 da / aE(a) (0 to a) = Ho
da / aE(a) (0 to a) = Ho  dt (0 to t) = t / tH,o
dt (0 to t) = t / tH,o
Notice how our equation follows the Newtonian form, with kinetic, gravitational, and total energy terms.
Plotting the three terms immediately gives us a feel for the behavior of the expansion:
[image]

 the dropping velocity matches the rising gravitational energy during the radiation/matter eras
the dropping velocity matches the rising gravitational energy during the radiation/matter eras
 the increasing velocity matches the dropping vacuum gravitational energy during the dark energy era.
the increasing velocity matches the dropping vacuum gravitational energy during the dark energy era.
Let's try to understand the gravitational terms, since they are the key to the behavior.
Returning to our upward moving rock at the surface of the expanding sphere, what is it's gravitational energy?
There are three contributions: from the matter, radiation and vacuum within the sphere.
The sphere's mass in matter is constant, so the rock's PE increases as -GMm,o/r giving - m,o / a.
m,o / a.
The sphere's mass in radiation decreases, so the rock's PE increases faster, as -GMr,o/r2 giving - r,o / a2.
r,o / a2.
The sphere's mass in vacuum increases, so the rock's PE actually falls, as -GMv,o/r × r3 giving - v,o a2.
v,o a2.
Since the total energy term (1 -  t,o) is fixed, the expansion velocity must mirror the gravitational terms.
t,o) is fixed, the expansion velocity must mirror the gravitational terms.
We can understand dark energy's accelerating expansion by considering its gravitational energy. [image]
Because larger spheres of vacuum have more mass, their gravitational energy is more negative
Hence, expansion is "downhill"  vacuum Universe's fall outwards.
vacuum Universe's fall outwards.
One often hears that dark energy "makes gravity repulsive", which refers to the force of gravity.
But force is really just the spatial gradient of energy: i.e. the gradient of the curve in the figure
This curve (- v,o a2) slopes downwards to the right, hence the force is outwards
v,o a2) slopes downwards to the right, hence the force is outwards
Notice, gravity is still "attractive", meaning its interaction still causes negative binding energy.
The time of coasting is at the top of the total binding energy curve, with gradient (force) zero.
 Matter force: d/da (-
Matter force: d/da (- m,o / a) =
m,o / a) =  m,o / a2 (backwards, inverse square, as expected)
m,o / a2 (backwards, inverse square, as expected)
 Vacuum force: d/da (-
Vacuum force: d/da (- v,o a2) = -2
v,o a2) = -2 v,o a (outwards, increasing with a).
v,o a (outwards, increasing with a).
Notice, kg for kg vacuum generates twice the force of matter, so coasting occurs before density equality.
We have:  m,o / ac2 - 2
m,o / ac2 - 2 v,o ac = 0
v,o ac = 0  ac = (
ac = ( m,o / 2
m,o / 2 v,o)1/3 = 0.57 (z = 0.75, t = 7 Gyr).
v,o)1/3 = 0.57 (z = 0.75, t = 7 Gyr).
One may wonder how a vacuum sphere can simply gain mass -- where does that mass come from?
Amazingly, the gravitational energy released as the sphere falls outwards makes new vacuum!.
[Strictly, this only happens for spheres larger than the Hubble radius. image ]
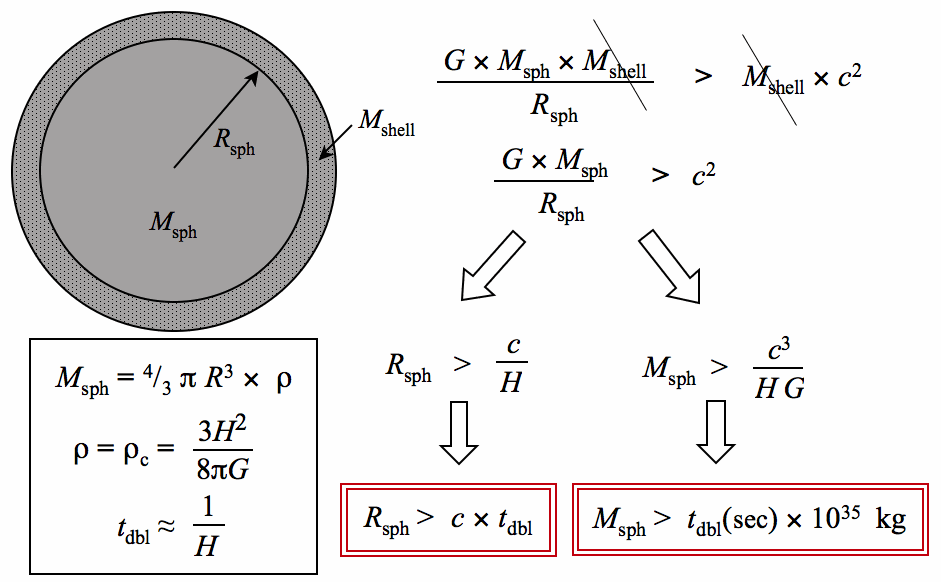
This is remarkable: gravity makes the very space into which the Universe is expanding!
(iv) Flat Models: Single Component
The special case of flat geometries with a single component are good pedagogical
models.
They also illustrate the behavior of the various "eras" in the multi-component models.
We use t = tH,o  da / aE(a) (from 0 to a); with E(a) containing just one term:
da / aE(a) (from 0 to a); with E(a) containing just one term:
|
1. Pure matter: |  m,o m,o | = 1; | a E(a) = [a-1]½ | t = (2/3) tH,o a3/2 | a = [3/2 t/tH,o]2/3
|
|
2. Pure radiation: |  r,o r,o | = 1; | a E(a) = [a-2]½ | t = (1/2) tH,o a2 | a = [2 t/tH,o]1/2 |
|
3. Pure vacuum: |  v,o v,o | = 1; | a E(a) = [a2]½ | t - to = tH,o loge a | a = e[(t - to)/tH,o] |
|
4. Pure curvature: |  k,o k,o | = 1; | a E(a) = [ k,o]½ k,o]½ | t = tH,o a | a = t / tH,o
|
These recover the forms from [sec 4e]: a  t2/3 , t1/2 , et for matter, radiation, vacuum.
t2/3 , t1/2 , et for matter, radiation, vacuum.
The pure curvature model is open (not flat), with simple linear expansion at all times.
Pure matter & curvature models are also called: Einstein-de Sitter & Milne [sec 6cvii]
Note: the coefficients are different from the concordance approximations
because the current  's aren't unity.
's aren't unity.
For a general equation of state parameter w, we have [sec 4e]:
a E(a) = [a-(1+3w)]½  t = 2/(3+3w) tH,o a(3+3w)/2
t = 2/(3+3w) tH,o a(3+3w)/2  a = [ (3 + 3w)/2 (t / tH,o) ]2/(3+3w)
a = [ (3 + 3w)/2 (t / tH,o) ]2/(3+3w)
you can quickly check this gives 1 & 2 above, for w = 0 and 1/3.
Note also that the flat model with w = -1/3 behaves like an open pure curvature (Milne) model.
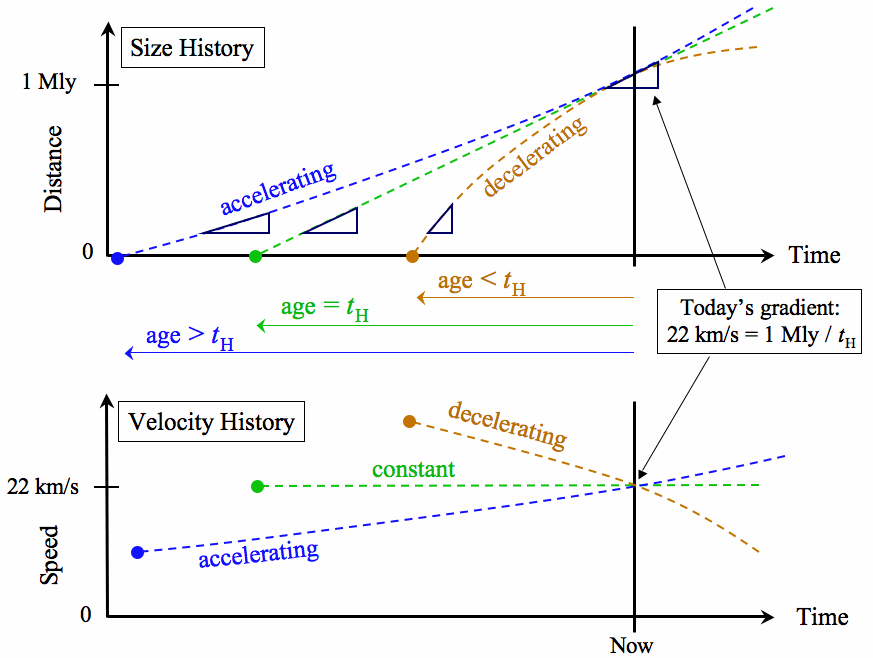
It is easy to find the current age of all these models: just set a = 1 and solve for t:
tage = 2/(3 + 3w) tH  2/3 tH , 1/2 tH , 2/3 tH , 1/2 tH ,  , tH for 1 - 4 above. , tH for 1 - 4 above.
|
Here I chose to use tH in place of tH,o since the relation for tage is true at all times.
As we suspected from the outset, tage  tH to within factors ~unity.
tH to within factors ~unity.
The exception is pure vacuum, with exponential expansion and infinite age (t  -
- as a
as a  0)
0)
In general, deceleration gives t < tH while acceleration gives t > tH [image]
Note that w = -1/3 separates ac-/de-celerating models, and hence tage greater/less than tH,o
For example, for flat matter (Einstein-de Sitter; w = 0) we have tage = 2/3 tH,o, a famous result.
By including a component with w < -1/3 (eg vacuum/lambda), then tage can get longer than tH,o
This was a key motivation for including  to solve tH,o < t
to solve tH,o < t (1930s), or tH,o < tGC (1990s).
(1930s), or tH,o < tGC (1990s).

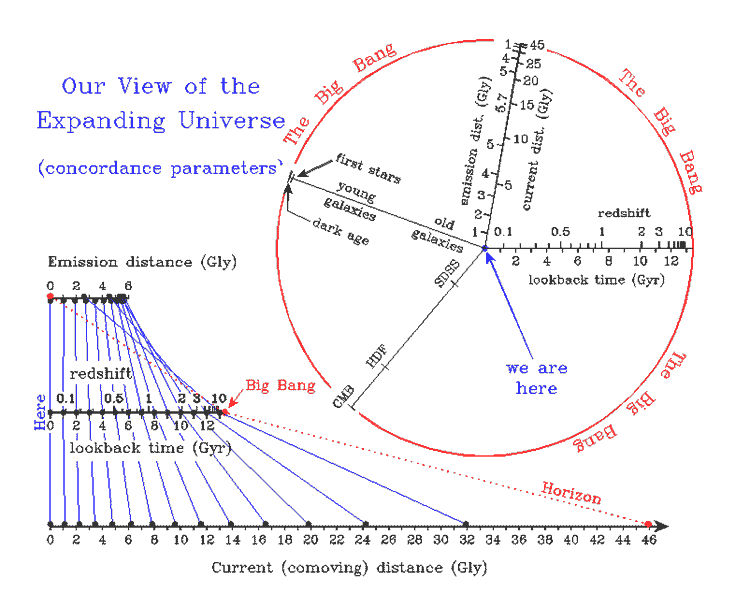
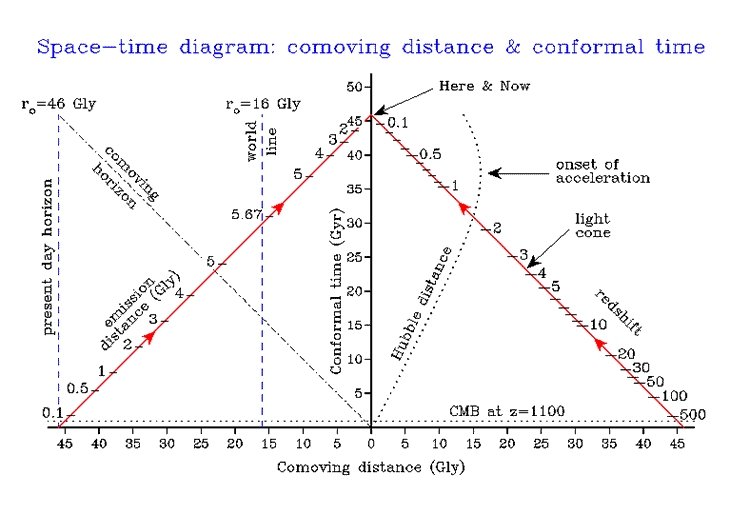
Some more a(t) curves for various models are given here [images]
(v) Flat Models: Matter + Vacuum
As it happens, it is possible to derive an analytic form for a flat universe with both  m and
m and  v
v
 Apart from a brief (~50 kyr) radiation period, this is basically the Universe we live in
[image]
Apart from a brief (~50 kyr) radiation period, this is basically the Universe we live in
[image]
As an exercise in integration, follow the analysis through to derive the following result:
a(t) = ( m,o /
m,o /  v,o)1/3 sinh2/3 [ (3/2)
v,o)1/3 sinh2/3 [ (3/2)  v,o1/2 (t / tH,o) ] = 0.712 × sinh2/3 (1.28 × t / tH,o)
v,o1/2 (t / tH,o) ] = 0.712 × sinh2/3 (1.28 × t / tH,o)
tage = (2/3) tH,o  v,o-1/2 sinh-1 [ (
v,o-1/2 sinh-1 [ ( v,o /
v,o /  m,o)1/2 ] = 0.78 × 1.27 tH = 0.993 tH,o
m,o)1/2 ] = 0.78 × 1.27 tH = 0.993 tH,o
which recovers an age conveniently close to tH,o  Ho-1
Ho-1
i.e. the decelerating & accelerating portions "average out" to approximate a constant expansion.
(the numbers here use:  v,o = 1 -
v,o = 1 -  m,o = 0.73)
m,o = 0.73)
(vi) Curved Models: Matter Only
For a long time (1960s-90s), curved matter models were favoured, justifying their inclusion here:
 open with infinite future; closed ending in a big crunch; or "critical", balanced between the two
open with infinite future; closed ending in a big crunch; or "critical", balanced between the two
There is, however, a more compelling reason: they are crucial in theories of galaxy formation
 while the real Universe seems to be globally flat, this is not true locally:
while the real Universe seems to be globally flat, this is not true locally:
 voids form from open regions; while stars/galaxies/clusters form from closed regions
voids form from open regions; while stars/galaxies/clusters form from closed regions
FRW curved matter models apply since local evolution is independent of the surroundings.
For a single component:  s =
s =  s,o a-3(1+w) and
a E(a) = [
s,o a-3(1+w) and
a E(a) = [ s,o a-(1+3w) + (1 -
s,o a-(1+3w) + (1 -  s,o)]1/2
s,o)]1/2
For w = 0 (matter) we have t(a) = tH,o  [
[  m,o a-1 + 1 -
m,o a-1 + 1 -  m,o ] -1/2 da (from 0 to a)
m,o ] -1/2 da (from 0 to a)
This has a parametric solution which uses a "development angle", 
The form of the solution depends on whether  m,o > 1 (closed) or
m,o > 1 (closed) or  m,o < 1 (open):
m,o < 1 (open):
For closed models with  = 0 to 2
= 0 to 2 , the Universe expands, halts, and recollapses in a cycloid:
, the Universe expands, halts, and recollapses in a cycloid:
a( ) = ½ amax (1 - cos ) = ½ amax (1 - cos  ) ) |
t( ) = ½ tH amax ( ) = ½ tH amax ( - sin - sin  ) / ( ) / ( m,o - 1)1/2 m,o - 1)1/2
|
which turns at a( ) = amax =
) = amax =  m,o / (
m,o / ( m,o - 1) and collapses at t(2
m,o - 1) and collapses at t(2 ) =
) =
 tH,o amax / (
tH,o amax / ( m,o - 1)1/2
m,o - 1)1/2
for example, if  m,o = 1.5, we have amax = 3 and tcrunch = 6.7 tH,o
m,o = 1.5, we have amax = 3 and tcrunch = 6.7 tH,o
For open models, the Universe expands forever with similar solution, with  = 0 to
= 0 to 
a( ) = ½ apar ( cosh ) = ½ apar ( cosh  - 1) - 1) |
t( ) = ½ tH,o apar ( sinh ) = ½ tH,o apar ( sinh  - -  ) / (1 - ) / (1 -  m,o)1/2 m,o)1/2
|
where apar =  m,o / (1 -
m,o / (1 -  m,o) plays the role of amax
m,o) plays the role of amax
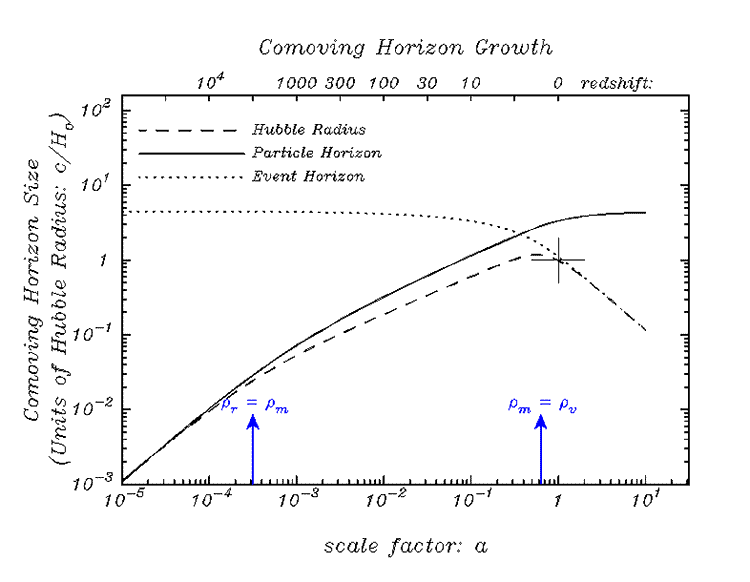
Newtonian Energy curves and the Expansion Histories for these matter models are shown here: [images]
(vii) Specific & Named World Models
This [figure] illustrates most classes of FRW world models.
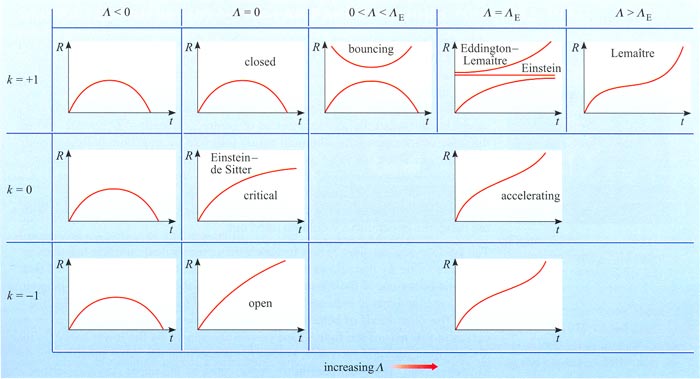
The two key parameters are
- curvature: closed (k = +1,
 k -ve), flat
(k = 0,
k -ve), flat
(k = 0,  k = 0), open (k = -1,
k = 0), open (k = -1,  k +ve)
k +ve)
- lambda:
 v: -ve (odd), 0 (simplest), +ve (what seems to be true).
v: -ve (odd), 0 (simplest), +ve (what seems to be true).
Full histories in linear time usually consider pressure-less matter along with vacuum.
This is because radiation quickly becomes irrelevant when
 r <
r <  m at t ~
50 kyr.
m at t ~
50 kyr.
Referring to the figures, the overall forms are:
 negative
negative  : unusual; simply adds to matter; all models recollapse
: unusual; simply adds to matter; all models recollapse
zero  : most discussed until recently; all decelerate; future depends only on curvature.
: most discussed until recently; all decelerate; future depends only on curvature.
closed recollapses; flat halts at 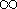 ; open expands forever. [images]
; open expands forever. [images]
positive  : early, matter dominates
: early, matter dominates  decelerates; late, vacuum dominates
decelerates; late, vacuum dominates  accelerates.
accelerates.
For a closed geometry, additional possibilities include (see below):
For  v <
v <  v,E
both collapse and "bounce" solutions exist
v,E
both collapse and "bounce" solutions exist
For  v
v 
 v,E vacuum repulsion and closed geometry nearly balance
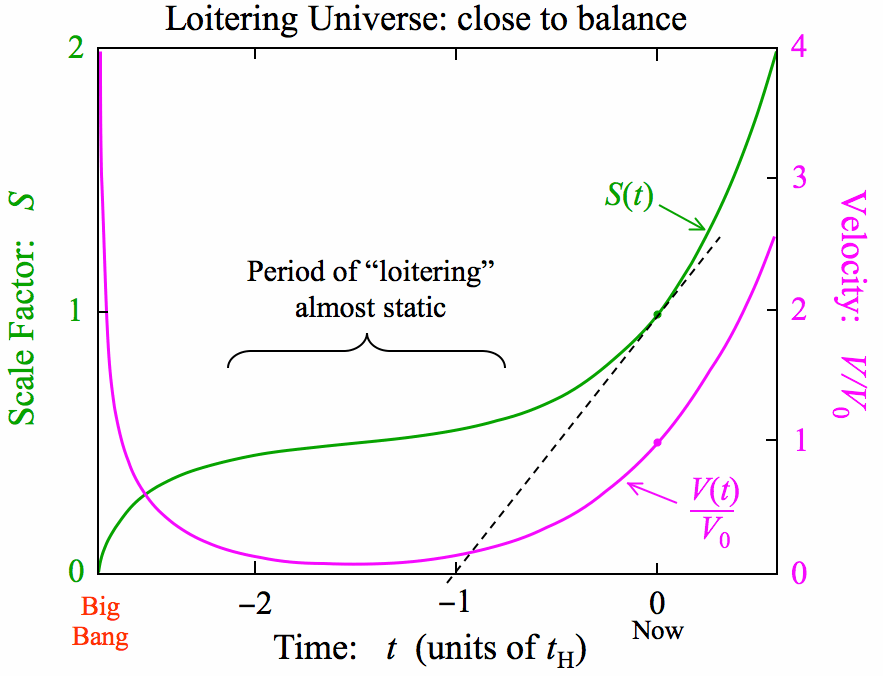
v,E vacuum repulsion and closed geometry nearly balance  long ~static periods.
long ~static periods.
Ages and futures for all these models can be nicely seen in these plots
[image]
Some of these models go by the names of those who advocated/studied them:
- Einstein-de Sitter: pure matter, flat; "Critical" classical model, between open & closed.
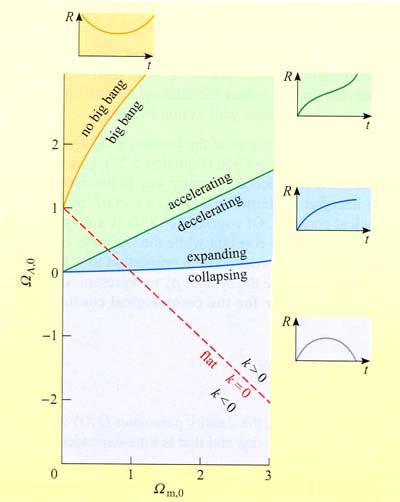
- de Sitter: pure vacuum, flat exponential expansion: a
 et; infinite past; H(a) = const [image]
et; infinite past; H(a) = const [image]
This model has renewed importance, since it is believed to occur during inflation.
It also describes the Steady State model, which needs H(a) = const.
- Einstein: His original model; curvature/matter/vacuum all balance to give static Universe.
pre-dated Hubble's discovery of expansion; doesn't fit above analysis since Ho = 0.
Instead, set da/dt = d2a/dt2 = 0 to find balance when:
 m+
m+  v -1 = 3/2 (
v -1 = 3/2 ( v
v m2)1/3
m2)1/3
The model is unstable to small deviations from balance.
- Eddington-Lemaitre: Two additional solutions to Einstein's model
Infinitely long static history before starting expansion
big bang in remote past, then expansion comes to gradual halt at infinite future.
- Lemaitre: close to Einstein model, with
 v (
v ( m) slightly below (above) the critical values.
m) slightly below (above) the critical values.
- Milne: pure curvature (empty, not even vacuum energy);
 k = +1, open, (k = -1).
k = +1, open, (k = -1).
Similar to  m/r/v << 1
m/r/v << 1  no de- ac-celeration: linear expansion a = t / tH; tage = tH
no de- ac-celeration: linear expansion a = t / tH; tage = tH
Milne's (incorrect) motivation: moving galaxies subject only to special relativity.
Some photos of many of the characters we've mentioned so far are shown here: [image]

 5 km s-1 Mpc-1
5 km s-1 Mpc-1

 = 0.73
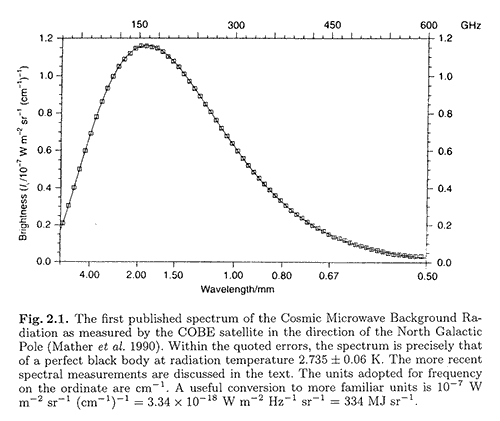
= 0.73  = 5.0 × 10-5 : CMB photons reveal an early hot phase
= 5.0 × 10-5 : CMB photons reveal an early hot phase = 3.4 × 10-5 : CNB neutrinos have been observed indirectly in the CMB power spectrum.
= 3.4 × 10-5 : CNB neutrinos have been observed indirectly in the CMB power spectrum.
 "The Concordance Model"
"The Concordance Model" 


 d
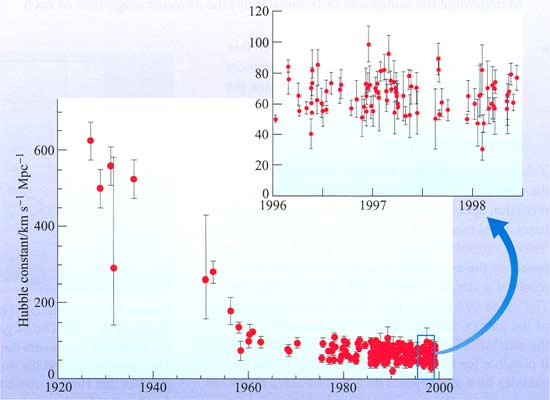
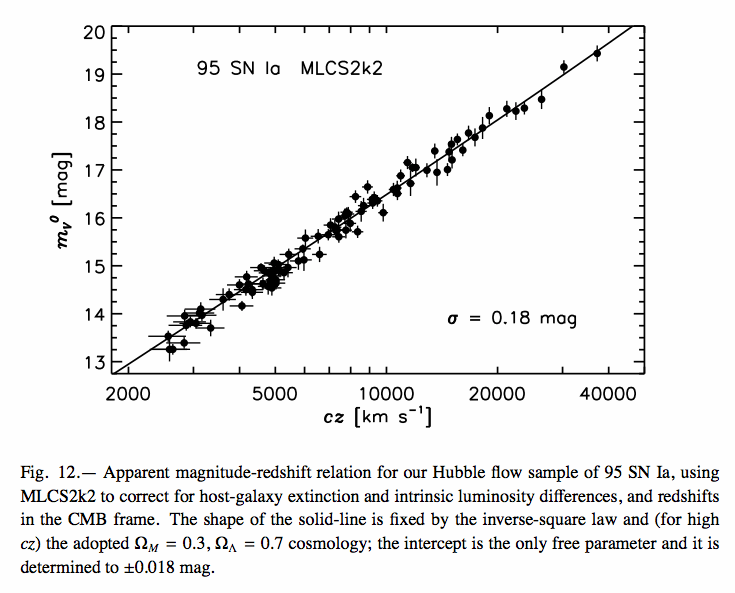
d 10% uncertainty in the gradient, Ho (see below)
10% uncertainty in the gradient, Ho (see below) " [
" [

 current age.
current age. =
= 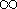 ), we have cosmic time:
), we have cosmic time: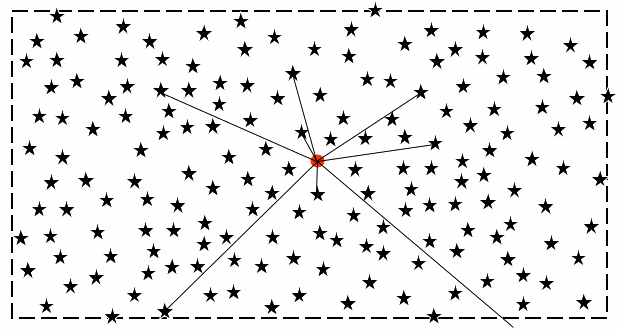
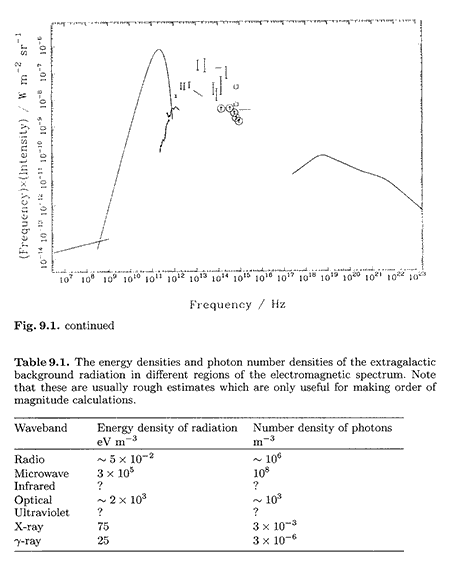
 ~ 108 Mpc-3;
~ 108 Mpc-3; 


 r2 =
r2 = 
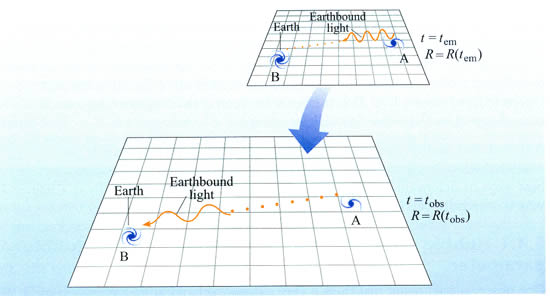
 obs/
obs/


 N fluctuations in # stars
N fluctuations in # stars
 T/T)CMB
T/T)CMB  0, one cannot infer
the future simply from
0, one cannot infer
the future simply from  3 is also possible.
3 is also possible.

 (loitering model)
(loitering model)
 /c is the radiation constant and NOT the scale factor!].
/c is the radiation constant and NOT the scale factor!].
 , each with particle/anti-particle pairs
, each with particle/anti-particle pairs 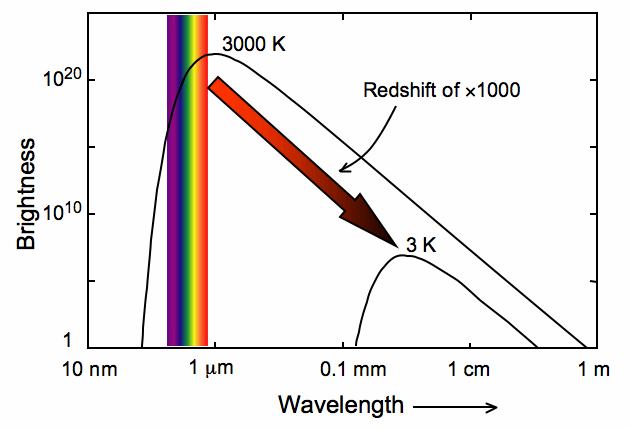
 systems:
systems: ,
,  ).
).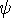 2
2

 2 in the Newtonian Poisson equation).
2 in the Newtonian Poisson equation). with 44 = 256 elements.
with 44 = 256 elements.